Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-29T13:55:08.510Z Has data issue: false hasContentIssue false
  • English
  • Français

Lifting n-Dimensional Galois Representations

Published online by Cambridge University Press:  20 November 2018

Spencer Hamblen
Spencer Hamblen*
Affiliation:
Department of Mathematics and Computer Science, McDaniel College, Westminster, MD, 21157-4390 e-mail:[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate the problem of deforming $n$-dimensional mod 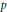$p$ Galois representations to characteristic zero. The existence of 2-dimensional deformations has been proven under certain conditions by allowing ramification at additional primes in order to annihilate a dual Selmer group. We use the same general methods to prove the existence of $n$-dimensional deformations.

We then examine under which conditions we may place restrictions on the shape of our deformations at 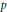$p$, with the goal of showing that under the correct conditions, the deformations may have locally geometric shape. We also use the existence of these deformations to prove the existence as Galois groups over $\mathbb{Q}$ of certain infinite subgroups of 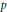$p$-adic general linear groups.

Keywords

11F80
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 60 , Issue 5 , October 2008 , pp. 1028 - 1049
Copyright
Copyright © Canadian Mathematical Society 2008

References

[1] Ash, A. andSinnott, W., An analogue of Serre's Conjecture for Galois Representations and Hecke Eigenclasses in the mod p cohomology of GL (n, ℤ). Duke Math. J. 105(2000), no. 1, 1–24, 2000.Google Scholar
[2] B, G.öckle and Khare, C., Mod l representations of arithmetic fundamental groups. I. Duke Math. J. 129(2005), no. 2, 339–369.Google Scholar
[3] Darmon, H., Diamond, F., and Taylor, R., Fermat's last theorem. In: Current Developments in Mathematics. International Press, Cambridge, MA, 1995, pp. 1–154.Google Scholar
[4] Diamond, F., An extension of Wiles’ results. In: Modular Forms and Fermat's Last Theorem. Springer-Verlag, New York, 1997.Google Scholar
[5] Fontaine, J.-M., Représentations p-adiques semi-stables. Astérique No. 223, 1994, pp. 113–184.Google Scholar
[6] Fontaine, J.-M. and Mazur, B., Geometric Galois representations. In: Elliptic Curves,Modular Forms, and Fermat's Last Theorem. International Press, Cambridge, MA, 1995, pp. 47–71.Google Scholar
[7] Mazur, B., Deforming Galois representations. In: Galois Groups over ℚ. Math. Sci. Res. Inst. Publ. 16. Springer, New York, 1989, pp. 385–437.Google Scholar
[8] Neukirch, J., Algebraic Number Theory. Grundlehren der MathematischenWissenschaften, 322. Springer-Verlag, Berlin, 1999.Google Scholar
[9] Neukirch, J., Schmidt, A., and Wingberg, K., Cohomology of Number Fields. Grundlehren der MathematischenWissenschaften 323, Springer-Verlag, Berlin, 2000.Google Scholar
[10] Perrin, B.-Riou, Théorie d’Iwasawa des représenttions p-adiques sur un corps local. Invent. Math. 115(1994), no. 1, 81–149.Google Scholar
[11] Ramakrishna, R., On a Variation of Mazur's Deformation Functor. Compos. Math. 87(1993), no. 3, 269–286.Google Scholar
[12] Ramakrishna, R., Lifting Galois representations. Invent. Math. 138(1999), no. 3, 537–562.Google Scholar
[13] Ramakrishna, R., Deforming Galois representations and the conjectures of Serre and Fontaine-Mazur. Ann. of Math. 156(2002), no. 1, 115–154.Google Scholar
[14] Raynaud, M., Schémas en groupes de type (p, p, … , p). Bull. Soc. Math. France 102(1974), 241–280.Google Scholar
[15] Ribet, K. andStein, W., Lectures on Serre's conjecture. In: Arithmetic Algebraic Geometry, IAS/Park CityMathematics Series. American Mathematical Society, Providence, RI, 1999.Google Scholar
[16] Serre, J.-P., Sur les représentations modulaires de degré 2 de Gal . Duke Math. J. 54(1987), no. 1, 179–230.Google Scholar
[17] Serre, J.-P., Abelian l-adic representations and elliptic curves. Research Notes in Mathematics 7. A K Peters,Wellesley, MA, 1998.Google Scholar
[18] Serre, J.-P., Galois Cohomology. Springer-Verlag, Berlin, 2002.Google Scholar
[19] Taylor, R., On icosahedral Artin representations. II. Amer. J. Math. 125(2003), 549–566.Google Scholar
[20] Thompson, J. G., PSL3 and Galois groups over Q. In: Proceedings of the Rutgers Group Theory Year, 1983–1984, Cambridge University Press, 1985, pp. 309–319.Google Scholar
[21] V, H.ölklein, GLn (q) as Galois group over the rationals. Math. Ann. 293(1992), no. 1, 163–176.Google Scholar
[22] Wiles, A., Modulare elliptic curves and Fermat's last theorem. Ann. of Math. 141(1995), no. 3, 443–551.Google Scholar
[23] Wilson, R. et al. ATLAS of Finite Group Representations, Version 1. http://web.mat.bham.ac.uk/atlas/v1.html.Google Scholar