Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-25T16:11:00.078Z Has data issue: false hasContentIssue false
  • English
  • Français

Kronecker Products and Local Joins of Graphs

Published online by Cambridge University Press:  20 November 2018

M. Farzan  and
D. A. Waller
M. Farzan
Affiliation:
University College of Swansea, Swansea, Great Britain
D. A. Waller
Affiliation:
University College of Swansea, Swansea, Great Britain
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

When studying the category 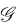raph of finite graphs and their morphisms, Ave can exploit the fact that this category has products, [we define these ideas in detail in § 2]. This categorical product of graphs is usually called their Kronecker product, though it has been approached by various authors in various ways and under various names, including tensor product, cardinal product, conjunction and of course categorical product (see for example [6; 7; 11 ; 14; 17 and 23]).

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 29 , Issue 2 , 01 April 1977 , pp. 255 - 269
Copyright
Copyright © Canadian Mathematical Society 1977

References

1. Biggs, N. L., Algebraic graph theory (Cambridge University Press, 1974).Google Scholar
2. Cvetkovic, D. M., The spectral method for determining the number of trees, Publ. Inst. Math. Beograd. 11 (25) (1971), 135–141.Google Scholar
3. Cvetkovic, D. M. Graphs and their spectra, Univ. Beograd. Publ. Elektrotehn. Fak. Ser. Mat. Fiz. 354-356 (1971), 1–50.Google Scholar
4. Dôrfler, W., Uber die X-summe von gerichteten Graphen, Arch. Math. 22 (1971), 24–36.Google Scholar
5. Dôrfler, W. Automorphism von X-summen von graphen, Czech. Math. J. 22 (97) (1971), 381–389.Google Scholar
6. Dôrfler, W. Zum Kroneckerprodukt von endlichen Graphen, Glasnik Mat. Ser. Ill 6 (16) (1971), 217–229.Google Scholar
7. Farzan, M., Matrix methods in graph theory, Thesis, University of Wales, Swansea 1974.Google Scholar
8. Finck, H.-J. and Grohmann, G., Vollstandiger Produkt, chromatische Zahl und characteriches Polynom regularer Graphen I, Wiss. Z. Techn. Hochsch. Ilmenau 11 (1965), 1–3.Google Scholar
9. Friedman, B., Eigenvalues of composite matrices, Proc. Camb. Phil. Soc. 57 (1961), 37–49.Google Scholar
10. Harary, F., Graph theory (Addison Wesley, 1969).Google Scholar
11. Hedetniemi, S. T., Homomorphisms of graphs and automata, Univ. of Michigan Technical Report, 03105–44-T, (1966).Google Scholar
12. Hemminger, R. L., The group of an X-join of graphs, J. Comb. Theory 5 (1968), 408–418.Google Scholar
13. Herrlich, H. and Strecker, G. E., Category theory (Allyn and Bacon, 1973).Google Scholar
14. Miller, D. J., The categorical product of graphs, Can. J. Math. 20 (1968), 1511–1521.Google Scholar
15. Sabidussi, G., Graph derivatives, Math. Zeitschr. 76 (1961), 385–401.Google Scholar
16. Sabidussi, G. The lexicographic product of graphs, Duke Math. J. 28 (1961), 573–578.Google Scholar
17. Sampathkumar, E., On tensor product graphs, J. Australian Math. Soc. 20 (Series A) (1975), 268–273.Google Scholar
18. Schwenk, A. J., Computing the characteristic polynomial of a graph, Springer Lecture Notes 406, 153–172.Google Scholar
19. Sumner, D. P., Graphs indecomposable with respect to the X-join, Discrete Math. 6 (1973), 281–298.Google Scholar
20. Waller, D. A., Eigenvalues of graphs and operations, in Combinatorics (eds. V. Mavron and T. McDonough), London Math. Soc. Lecture Notes 13, Cambridge U.P., 177–183.Google Scholar
21. Waller, D. A. Regular eigenvalues of graphs and enumeration of spanning trees, Proc. Colloquio Internazionale sulle Teorie Combinatorie, Rome 1973, I, 313–320.Google Scholar
22. Waller, D. A. Double covers of graphs, Bull. Australian Math. Soc. 14 (1976), 233–248.Google Scholar
23. Weichsel, P. M., The Kronecker product of graphs, Proc. Amer. Math. Soc. 13 (1962), 47–52.Google Scholar