Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T11:48:07.866Z Has data issue: false hasContentIssue false
  • English
  • Français

The Iteration of Certain Arithmetic Functions

Published online by Cambridge University Press:  20 November 2018

Ivan Niven
Ivan Niven*
Affiliation:
University of Oregon
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

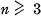1. Introduction. For define C(n) to be the integer j such that 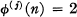, where 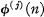denotes the j th iterate of the Euler ø-function. Define C(l) = C(2) = 0. This function has been studied by S. S. Pillai [1], with the notation R(n) for 1 + C(n) if , and R(l) = 0. H. Shapiro [2] has also investigated this function, proving the basic relations

(1)

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 2 , 1950 , pp. 406 - 408
Copyright
Copyright © Canadian Mathematical Society 1950

References

[1] Pillai, S. S., On a function connected with ø(n), Bull. Amer. Math. Soc, vol. 35 (1929), 837–841.Google Scholar
[2] Shapiro, Harold, An arithmetic function arising from the ø function, Amer. Math. Monthly, vol. 50 (1943), 18–30.Google Scholar