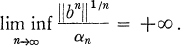Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Esterle, J.
1983.
Radical Banach Algebras and Automatic Continuity.
Vol. 975,
Issue. ,
p.
4.


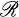 possesses bounded approximate identities then for every sequence (
possesses bounded approximate identities then for every sequence ( such that
such that