Soit 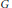$G$ un groupe réductif connexe défini sur un corps 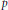$p$-adique $F$ et 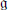$\mathfrak{g}$ son algèbre de Lie. Les intégrales orbitales pondérées sur $\mathfrak{g}\left( F \right)$ sont des distributions 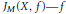${{J}_{M}}\left( X,\,f \right)-f$ est une fonction test—indexées par les sous-groupes de Lévi 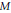$M$ de 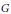$G$ et les éléments semi-simples réguliers $X\,\in \,\mathfrak{m}\left( F \right)\,\cap \,{{\mathfrak{g}}_{\text{reg}}}$. Leurs analogues sur 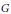$G$ sont les principales composantes du côté géométrique des formules des traces locale et globale d’Arthur.

Si $M=G$, on retrouve les intégrales orbitales invariantes qui, vues comme fonction de 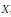$X$, sont borńees sur $\mathfrak{m}\left( F \right)\,\cap \,{{\mathfrak{g}}_{\text{reg}}}$ : c’est un résultat bien connu de Harish-Chandra. Si 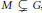$M\subsetneq G$, les intégrales orbitales pondérées explosent au voisinage des éléments singuliers. Nous construisons dans cet article de nouvelles intégrales orbitales pondérées $J_{M}^{b}\left( X,f \right)$, égales à ${{J}_{M}}\left( X,f \right)$ à un terme correctif près, qui tout en conservant les principales propriétés des précédentes (comportement par conjugaison, développement en germes, etc.) restent borńees quand $X$ parcourt $\mathfrak{m}\left( F \right)\,\cap \,{{\mathfrak{g}}_{\text{reg}}}$. Nous montrons également que les intégrales orbitales pondérées globales, associées à des éléments semi-simples réguliers, se décomposent en produits de ces nouvelles intégrales locales.