Let 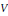$V$ be an analytic variety in some open set in 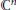${{\mathbb{C}}^{n}}$ which contains the origin and which is purely 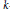$k$-dimensional. For a curve $\gamma $ in 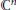${{\mathbb{C}}^{n}}$, defined by a convergent Puiseux series and satisfying 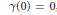$\gamma (0)\,=\,0$, and 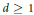$d\,\ge \,1$, define 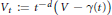${{V}_{t}}\,:=\,{{t}^{-d}}\,\left( V\,-\,\gamma \left( t \right) \right)$. Then the currents defined by ${{V}_{t}}$ converge to a limit current 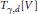${{T}_{\gamma ,d}}\left[ V \right]$ as $t$ tends to zero. 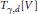${{T}_{\gamma ,d}}\left[ V \right]$ is either zero or its support is an algebraic variety of pure dimension 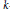$k$ in 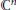${{\mathbb{C}}^{n}}$. Properties of such limit currents and examples are presented. These results will be applied in a forthcoming paper to derive necessary conditions for varieties satisfying the local Phragmén-Lindelöf condition that was used by Hörmander to characterize the constant coefficient partial differential operators which act surjectively on the space of all real analytic functions on 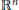${{\mathbb{R}}^{n}}$.