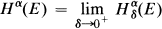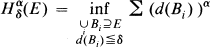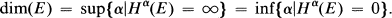Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cutler, C.D
and
Dawson, D.A
1989.
Estimation of dimension for spatially distributed data and related limit theorems.
Journal of Multivariate Analysis,
Vol. 28,
Issue. 1,
p.
115.
Cutler, C. D.
1990.
Connecting ergodicity and dimension in dynamical systems.
Ergodic Theory and Dynamical Systems,
Vol. 10,
Issue. 3,
p.
451.
Cutler, C. D.
1991.
Some results on the behavior and estimation of the fractal dimensions of distributions on attractors.
Journal of Statistical Physics,
Vol. 62,
Issue. 3-4,
p.
651.
Waymire, Edward C.
and
Williams, Stanley C.
1994.
A general decomposition theory for random cascades.
Bulletin of the American Mathematical Society,
Vol. 31,
Issue. 2,
p.
216.
Hu, Xiaoyu
and
Taylor, S. James
1994.
Fractal properties of products and projections of measures in ℝd.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 115,
Issue. 3,
p.
527.
Cutler, Colleen D.
1995.
Strong and weak duality principles for fractal dimension in Euclidean space.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 118,
Issue. 3,
p.
393.
Waymire, Edward
and
Williams, Stanley
1996.
A cascade decomposition theory with applications to Markov and exchangeable cascades.
Transactions of the American Mathematical Society,
Vol. 348,
Issue. 2,
p.
585.
Williams, Stanley
1996.
Transfinite multifractal dimension spectrums.
Transactions of the American Mathematical Society,
Vol. 348,
Issue. 10,
p.
4043.
Waymire, Edward C.
and
Williams, Stanley C.
1997.
Classical and Modern Branching Processes.
Vol. 84,
Issue. ,
p.
305.
Arbeiter, Matthias
1997.
Fractal random measures generated by time continuous branching and diffusion.
Stochastics and Stochastic Reports,
Vol. 60,
Issue. 1-2,
p.
107.
Vojak, Robert
1997.
On the influence of coverings in multifractal analysis.
Comptes Rendus de l'Académie des Sciences - Series I - Mathematics,
Vol. 325,
Issue. 11,
p.
1163.
Falconer, K. J.
and
O'Neil, T. C.
1999.
Convolutions and the Geometry of Multifractal Measures.
Mathematische Nachrichten,
Vol. 204,
Issue. 1,
p.
61.
Falconer, K. J.
and
O'Neil, T. C.
1999.
Convolutions and the Geometry of Multifractal Measures.
Mathematische Nachrichten,
Vol. 204,
Issue. 1,
p.
61.
Yu, Jinghu
and
Fan, Wentao
2000.
FRACTAL PROPERTIES OF STATISTICALLY SELF-SIMILAR SETS.
Acta Mathematica Scientia,
Vol. 20,
Issue. 2,
p.
256.
2001.
Multifractals.
Kenneth, J. Falconer
2002.
Handbook of Measure Theory.
p.
1037.
Cutler, Colleen D.
and
Hall, Peter
2004.
Encyclopedia of Statistical Sciences.
Cutler, Colleen D.
and
Hall, Peter
2005.
Encyclopedia of Statistical Sciences.
Riedi, Rudolf
2009.
Scaling, Fractals and Wavelets.
p.
139.
Dai, Meifeng
2009.
Multifractal analysis of a measure of multifractal exact dimension.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 70,
Issue. 2,
p.
1069.