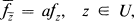Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hengartner, Walter
and
Schober, Glenn
1988.
Complex Analysis.
p.
87.
Abu-Muhanna, Yusuf
1999.
On harmonic univalent functions.
Complex Variables, Theory and Application: An International Journal,
Vol. 39,
Issue. 4,
p.
341.
Grigoryan, Armen
and
Nowak, Maria
2000.
Estimates of integral means of harmonic mappings.
Complex Variables, Theory and Application: An International Journal,
Vol. 42,
Issue. 2,
p.
151.
Dorff, Michael
2001.
Convolutions of planar harmonic convex mappings.
Complex Variables, Theory and Application: An International Journal,
Vol. 45,
Issue. 3,
p.
263.
Majchrzak, W.
2001.
Harmonic Univalent Mappings into a Half-Plane with Nonreal Vertical Slits.
Journal of Mathematical Analysis and Applications,
Vol. 255,
Issue. 2,
p.
519.
Wang, Xiao-Tian
Liang, Xiang-Qian
and
Zhang, Yu-Lin
2003.
On harmonic typically real mappings.
Journal of Mathematical Analysis and Applications,
Vol. 277,
Issue. 2,
p.
533.
Duren, Peter
2004.
Harmonic Mappings in the Plane.
AbdulHadi, Z.
Muhanna, Y. Abu
and
Khuri, S.
2006.
On some properties of solutions of the biharmonic equation.
Applied Mathematics and Computation,
Vol. 177,
Issue. 1,
p.
346.
Grigoryan, A.
and
Szapiel, W.
2007.
An existence theorem for harmonic homeomorphisms with a given range and some convergence theorems.
Complex Variables and Elliptic Equations,
Vol. 52,
Issue. 4,
p.
341.
Muhanna, Yusuf
2008.
On univalence of biharmonic maps.
Complex Variables and Elliptic Equations,
Vol. 53,
Issue. 8,
p.
745.
Abdulhadi, Z.
and
Abu Muhanna, Y.
2008.
Landau's theorem for biharmonic mappings.
Journal of Mathematical Analysis and Applications,
Vol. 338,
Issue. 1,
p.
705.
Abdulhadi, Zayid
2009.
On the univalence of functions with logharmonic laplacian.
Applied Mathematics and Computation,
Vol. 215,
Issue. 5,
p.
1900.
Abdulhadi, Z.
Abumuhanna, Y.
and
Ali, R.M.
2012.
Landau’s theorem for functions with logharmonic Laplacian.
Applied Mathematics and Computation,
Vol. 218,
Issue. 12,
p.
6798.
Dorff, Michael
Nowak, Maria
and
Wołoszkiewicz, Magdalena
2012.
Convolutions of harmonic convex mappings.
Complex Variables and Elliptic Equations,
Vol. 57,
Issue. 5,
p.
489.
Liu, Zhi-Hong
and
Li, Ying-Chun
2013.
The Properties of a New Subclass of Harmonic Univalent Mappings.
Abstract and Applied Analysis,
Vol. 2013,
Issue. ,
p.
1.
Li, Liulan
and
Ponnusamy, Saminathan
2013.
Solution to an open problem on convolutions of harmonic mappings.
Complex Variables and Elliptic Equations,
Vol. 58,
Issue. 12,
p.
1647.
Boyd, Z.
Dorff, M.
Nowak, M.
Romney, M.
and
Wołoszkiewicz, M.
2014.
Univalency of convolutions of harmonic mappings.
Applied Mathematics and Computation,
Vol. 234,
Issue. ,
p.
326.
El Hajj, Layan
2017.
On the univalence of polyharmonic mappings.
Journal of Mathematical Analysis and Applications,
Vol. 452,
Issue. 2,
p.
871.
Beig, Subzar
and
Ravichandran, V.
2017.
Directional Convexity of Harmonic Mappings.
Bulletin of the Malaysian Mathematical Sciences Society,
Liu, ZhiHong
and
Ponnusamy, Saminathan
2017.
Univalency of Convolutions of Univalent Harmonic Right Half-Plane Mappings.
Computational Methods and Function Theory,
Vol. 17,
Issue. 2,
p.
289.