Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T18:41:36.919Z Has data issue: false hasContentIssue false
  • English
  • Français

A group-theoretic generalization of the p-adic local monodromy theorem

Part of: Algebraic number theory: local and $p$-adic fields Lie algebras and Lie superalgebras Linear algebraic groups and related topics

Published online by Cambridge University Press:  29 June 2021

Shuyang Ye
Shuyang Ye*
Affiliation:
Department of Mathematics, East China Normal University, Shanghai, China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

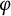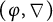Let G be a connected reductive group over a p-adic number field F. We propose and study the notions of G- $\varphi $ -modules and G- $(\varphi ,\nabla )$ -modules over the Robba ring, which are exact faithful F-linear tensor functors from the category of G-representations on finite-dimensional F-vector spaces to the categories of 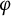$\varphi $ -modules and 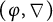$(\varphi ,\nabla )$ -modules over the Robba ring, respectively, commuting with the respective fiber functors. We study Kedlaya’s slope filtration theorem in this context, and show that G- $(\varphi ,\nabla )$ -modules over the Robba ring are “G-quasi-unipotent,” which is a generalization of the p-adic local monodromy theorem proved independently by Y. André, K. S. Kedlaya, and Z. Mebkhout.

Keywords

Robba ringp-adic local monodromy theoremreductive groups

MSC classification

Primary: 11S85: Other nonanalytic theory
Secondary: 20G25: Linear algebraic groups over local fields and their integers 17B45: Lie algebras of linear algebraic groups
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 5 , October 2022 , pp. 1450 - 1485
Check for updates
Copyright
© Canadian Mathematical Society 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This paper is partially supported by a research grant from Shanghai Key Laboratory of PMMP 18dz2271000.

References

André, Y., Filtrations de type Hasse–Arf et monodromie $p$ -adique . Invent. Math. 148(2002), 285–317.Google Scholar
Anschütz, J., Reductive group schemes over the Fargues–Fontaine curve. Math. Ann. 374(2019), 1219–1260.Google Scholar
Berger, L., Représentations  $p$ -adiques et équations différentielles . Invent. Math. 148(2002), 219–284.CrossRefGoogle Scholar
Conrad, B., Gabber, O., and Prasad, G., Pseudo-reductive groups. 2nd ed., New Mathematical Monographs, 26, Cambridge University Press, Cambridge, 2015.CrossRefGoogle Scholar
Crew, R., Finiteness theorems for the cohomology of an overconvergent isocrystal on a curve. Ann. Sci. École Norm. Sup. 31(1998), 717–763.CrossRefGoogle Scholar
Dat, J.-F., Orlik, S., and Rapoport, M., Period domains over finite and p-adic fields. Cambridge Tracts in Mathematics, 183, Cambridge University Press, Cambridge, 2010.CrossRefGoogle Scholar
Deligne, P. and Milne, J., Tannakian categories. In: Hodge cycles, motives, and Shimura varieties, Lecture Notes in Mathematics, 900, Springer, 1982, pp. 101–228.CrossRefGoogle Scholar
Demazure, M. and Gabriel, P., Groupes algébriques. Tome I: Géométrie algébrique, généralités, groupes commutatifs. Masson, Paris-Amsterdam, 1970.Google Scholar
Kedlaya, K. S., A  $p$ -adic local monodromy theorem . Ann. Math. 160(2004), 93–184.CrossRefGoogle Scholar
Kedlaya, K. S., Slope filtrations revisited. Doc. Math. 10(2005), 447–525.Google Scholar
Kedlaya, K. S., Slope filtrations for relative Frobenius. Astérisque 317(2008), 259–301.Google Scholar
Kedlaya, K. S., p-Adic differential equations. Cambridge Studies in Advanced Mathematics, 125, Cambridge University Press, Cambridge, 2010.CrossRefGoogle Scholar
Kedlaya, K. S., Notes on isocrystals. Preprint, 2018. arXiv:1606.01321.Google Scholar
Kottwitz, R., Isocrystals with additional structures. Compos. Math. 56(1985), 201–220.Google Scholar
Liu, R., Slope filtrations in families. J. Inst. Math. Jussieu 12(2013), 249–296.CrossRefGoogle Scholar
Marmora, A., Facteurs epsilon  $p$ -adiques . Compos. Math. 144(2008), 439–483.Google Scholar
Mebkhout, Z., Analogue  $p$ -adique du théorème de turrittin et le théorème de la monodromie  $p$ -adique . Invent. Math. 148(2002), 319–351.CrossRefGoogle Scholar
Milne, J., Algebraic groups. Cambridge Studies in Advanced Mathematics, 170, Cambridge University Press, Cambridge, 2017.CrossRefGoogle Scholar
Serre, J.-P., Galois cohomology. Corrected second printing edition, Springer Monographs in Mathematics, Springer-Verlag, Berlin-Heidelberg, 2002.Google Scholar
Springer, T. A., Linear algebraic groups. 2nd ed., Modern Birkhäuser Classics, Birkhäuser, Boston, 1998.CrossRefGoogle Scholar
Steinberg, R., Regular elements of semisimple algebraic groups. Inst. Hautes Études Sci. Publ. Math. 25(1965), 49–80.CrossRefGoogle Scholar
Tsuzuki, N., The overconvergence of morphisms of etale  $\varphi \hbox{--} \nabla$ -spaces on a local field . Compos. Math. 103(1996), 227–239.Google Scholar
Tsuzuki, N., Finite local monodromy of overconvergent unit-root  $F$ -isocrystals on a curve . Amer. J. Math. 120(1998), 1165–1190.CrossRefGoogle Scholar
Tsuzuki, N., Slope filtration of quasi-unipotent overconvergent  $F$ -isocrystals . Ann. Inst. Fourier 48(1998), 379–412.CrossRefGoogle Scholar
Ziegler, P., Graded and filtered fiber functors on Tannakian categories. J. Inst. Math. Jussieu 14(2015), 87–130.CrossRefGoogle Scholar