Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mukherjee, Goutam
1995.
Equivariant cobordism of Grassmann and flag manifolds.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 105,
Issue. 4,
p.
381.
Mukherjee, Goutam
and
Sankaran, Parameswaran
1998.
Elementary abelian 2-group actions on flag manifolds and applications.
Proceedings of the American Mathematical Society,
Vol. 126,
Issue. 2,
p.
595.
Mondal, Arghya
and
Sankaran, Parameswaran
2016.
Degrees of maps between locally symmetric spaces.
Bulletin des Sciences Mathématiques,
Vol. 140,
Issue. 5,
p.
488.
Mukherjee, Goutam
and
Sankaran, Parameswaran
2019.
Algebraic topology in India.
Indian Journal of Pure and Applied Mathematics,
Vol. 50,
Issue. 3,
p.
705.


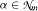 (respectively α є Ω
(respectively α є Ω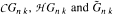 which are oriented boundaries as well as those which represent non-torsion elements in Ω
which are oriented boundaries as well as those which represent non-torsion elements in Ω