Published online by Cambridge University Press: 20 November 2018
Iwasawa's classical asymptotical formula relates the orders of the  $p$-parts
$p$-parts 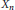 ${{X}_{n}}$ of the ideal class groups along a
${{X}_{n}}$ of the ideal class groups along a 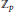 ${{\mathbb{Z}}_{p}}$-extension
${{\mathbb{Z}}_{p}}$-extension 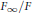 ${{F}_{\infty }}/F$ of a number field
${{F}_{\infty }}/F$ of a number field  $F$ to Iwasawa structural invariants
$F$ to Iwasawa structural invariants  $\lambda $ and
$\lambda $ and 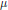 $\mu $ attached to the inverse limit
$\mu $ attached to the inverse limit 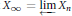 ${{X}_{\infty }}=\underleftarrow{\lim }\,{{X}_{n}}$. It relies on “good” descent properties satisfied by
${{X}_{\infty }}=\underleftarrow{\lim }\,{{X}_{n}}$. It relies on “good” descent properties satisfied by 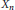 ${{X}_{n}}$. If
${{X}_{n}}$. If  $F$ is abelian and
$F$ is abelian and  ${{F}_{\infty }}$ is cyclotomic, it is known that the
${{F}_{\infty }}$ is cyclotomic, it is known that the  $p$-parts of the orders of the global units modulo circular units
$p$-parts of the orders of the global units modulo circular units 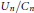 ${{U}_{n}}/{{C}_{n}}$ are asymptotically equivalent to the
${{U}_{n}}/{{C}_{n}}$ are asymptotically equivalent to the  $p$-parts of the ideal class numbers. This suggests that these quotients
$p$-parts of the ideal class numbers. This suggests that these quotients 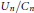 ${{U}_{n}}/{{C}_{n}}$, so to speak unit class groups, also satisfy good descent properties. We show this directly, i.e., without using Iwasawa's Main Conjecture.
${{U}_{n}}/{{C}_{n}}$, so to speak unit class groups, also satisfy good descent properties. We show this directly, i.e., without using Iwasawa's Main Conjecture.