“Co-Frobenius” coalgebras were introduced as dualizations of Frobenius algebras. We previously showed that they admit left-right symmetric characterizations analogous to those of Frobenius algebras. We consider the more general quasi-co-Frobenius 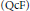$\left( \text{QcF} \right)$ coalgebras. The first main result in this paper is that these also admit symmetric characterizations: a coalgebra is 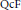$\text{QcF}$ if it is weakly isomorphic to its (left, or right) rational dual 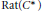$\text{Rat}\left( {{C}^{*}} \right)$ in the sense that certain coproduct or product powers of these objects are isomorphic. Fundamental results of Hopf algebras, such as the equivalent characterizations of Hopf algebras with nonzero integrals as left (or right) co-Frobenius, 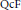$\text{QcF}$, semiperfect or with nonzero rational dual, as well as the uniqueness of integrals and a short proof of the bijectivity of the antipode for such Hopf algebras all follow as a consequence of these results. This gives a purely representation theoretic approach to many of the basic fundamental results in the theory of Hopf algebras. Furthermore, we introduce a general concept of Frobenius algebra, which makes sense for infinite dimensional and for topological algebras, and specializes to the classical notion in the finite case. This will be a topological algebra $A$ that is isomorphic to its complete topological dual 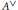${{A}^{\vee }}$. We show that $A$ is a (quasi)Frobenius algebra if and only if $A$ is the dual 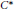${{C}^{*}}$ of a (quasi)co-Frobenius coalgebra $C$. We give many examples of co-Frobenius coalgebras and Hopf algebras connected to category theory, homological algebra and the newer $q$-homological algebra, topology or graph theory, showing the importance of the concept.