Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T05:47:03.240Z Has data issue: false hasContentIssue false
  • English
  • Français

Galois Module Structure of Ambiguous Ideals in Biquadratic Extensions

Published online by Cambridge University Press:  20 November 2018

G. Griffith Elder
G. Griffith Elder*
Affiliation:
Department of Mathematics, University of Nebraska at Omaha, Omaha, Nebraska 68132, U.S.A. email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 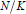$N/K$ be a biquadratic extension of algebraic number fields, and 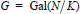$G\,=\,\text{Gal(}N/K\text{)}$. Under a weak restriction on the ramification filtration associated with each prime of 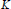$K$ above 2, we explicitly describe the 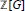$\mathbb{Z}\text{ }[G]\text{ }$-module structure of each ambiguous ideal of $N$. We find under this restriction that in the representation of each ambiguous ideal as a 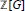$\mathbb{Z}\text{ }[G]\text{ }$-module, the exponent (or multiplicity) of each indecomposable module is determined by the invariants of ramification, alone.

For a given group, 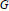$G$, define ${{S}_{G}}$ to be the set of indecomposable 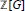$\mathbb{Z}\text{ }[G]\text{ }$-modules, $M$, such that there is an extension, 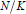$N/K$, for which $G\cong \text{Gal(}N/K\text{)}$, and $M$ is a 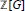$\mathbb{Z}\text{ }[G]\text{ }$-module summand of an ambiguous ideal of $N$. Can 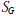${{S}_{G}}$ ever be infinite? In this paper we answer this question of Chinburg in the affirmative.

Keywords

11R3311S1520C32Galois module structureWild ramification
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 50 , Issue 5 , 01 October 1998 , pp. 1007 - 1047
Copyright
Copyright © Canadian Mathematical Society 1998

References

1. Bley, W. and Burns, D., Über Arithmetische Assoziierte Ordnungen. J. Number Theory (2) 58(1996), 361–387.Google Scholar
2. Burns, D., Factorisability and wildly ramified Galois extensions. Ann. Inst. Fourier (Grenoble) 41(1991), 393–430.Google Scholar
3. Dieterich, E., Representation types of group rings over complete discrete valuation rings. In: Integral representations and applications, Olberwolfach, 1980. Lecture Notes inMath., Springer, Berlin, New York, 1981. 369–389.Google Scholar
4. Cohen, H., A course in computational algebraic number theory. Springer-Verlag.Graduate Texts inMath. 138, Berlin, Heidelberg, 1993.Google Scholar
5. Curtis, C.W. and Reiner, I., Methods of Representation Theory. Wiley, New York 1981.Google Scholar
6. Elder, G.G. and Madan, M.L., Galois module structure of integers in wildly ramified cyclic extensions. J. Number Theory (2) 47(1994), 138–174.Google Scholar
7. Elder, G.G., Galois module structure of integers in wildly ramified Cp ð Cp extensions. Canad. J. Math. (4) 49(1997), 722–735.Google Scholar
8. Elder, G.G., Galois module structure of integers in wildly ramified cyclic extensions of degree p2. Ann. Inst. Fourier (Grenoble) (3) 45(1995), 625–647. errata ibid. (2) 48(1998), 609–610.Google Scholar
9. Fröhlich, A., Galois Module Structure of Algebraic Integers. In: Ergebnisse der Mathematik und ihrer Grenzgebiete 3, Folge, Bd. 1, Springer-Verlag, Berlin, Heidelberg, New York, 1983.Google Scholar
10. Fröhlich, A. and Taylor, M.J., Algebraic Number Theory. Camb. Stu. Adv. Math. 27, Cambridge Univ. Press, 1991.Google Scholar
11. Jakobinski, H., Genera and Decompositions of Lattices over Orders. Acta.Math. 121(1968), 1–29.Google Scholar
12. Leopoldt, H.W., Über die Hauptordnung der ganzen Elemente eines abelschen Zahlkörpers. J. Reine Angew. Math. 201(1959), 119–149.Google Scholar
13. Martel, B., Sur l’anneau des entiers d’une extension biquadratique d’un corps 2-adique. C. R. Acad. Sci. Paris 278(1974), 117–120.Google Scholar
14. Maus, E., Arithmetisch disjunkte Körper. J. Reine Angew. Math. 226(1967), 184–203.Google Scholar
15. Miyata, Y., On the module structure of a p-extension over a p-adic number field. Nagoya Math. J. 77(1980), 13–23.Google Scholar
16. Nazarova, L.A., Integral representations of Klein's four-group. SovietMath. Dokl. 2(1961), 1304.ndash;1307; English Translation.Google Scholar
17. Nazarova, L.A., Representation of a Tetrad. Math. USSR-Izv. (6) 1(1967), 1305.ndash;1321; English Translation.Google Scholar
18. Noether, E., Normalbasis bei Körpern ohne höhere Verzweigung. J. Reine Angew. Math. 167(1932), 147–152.Google Scholar
19. Rzedowski-Calderón, M., Villa, G.D.-Salvador and Madan, M.L., Galois module structure of rings of integers. Math. Z. 204(1990), 401–424.Google Scholar
20. Sen, S., On automorphisms of local fields. Ann. of Math. (2) 90(1969), 33–46.Google Scholar
21. Serre, J-P., Local fields. Graduate Texts in Math. 67, Springer-Verlag, Berlin, Heidelberg, New York, 1979.Google Scholar
22. Vostokov, S.V., Ideals of an abelian p-extension of a local field as Galois modules. Zap. Nauchn. Sem. Leningrad. Otdel. Mat. Inst. Akad. Nauk SSSR 57(1976), 64–84.Google Scholar
23. Wiegand, R., Cancellation over Commutative Rings of Dimension One and Two. J.Algebra. (2) 88(1984), 438–459.Google Scholar
24. Wyman, B., Wildly ramified gamma extensions. Amer. J.Math. 91(1969), 135–152.Google Scholar