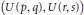Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yamana, Shunsuke
2014.
L-functions and theta correspondence for classical groups.
Inventiones mathematicae,
Vol. 196,
Issue. 3,
p.
651.
Sun, Binyong
and
Zhu, Chen-Bo
2014.
Conservation relations for local theta correspondence.
Journal of the American Mathematical Society,
Vol. 28,
Issue. 4,
p.
939.
Bao, YiXin
and
Sun, BinYong
2017.
Coincidence of algebraic and smooth theta correspondences.
Representation Theory of the American Mathematical Society,
Vol. 21,
Issue. 16,
p.
458.
Sun, Binyong
and
Zhu, Chen-Bo
2017.
Representation Theory, Number Theory, and Invariant Theory.
Vol. 323,
Issue. ,
p.
587.
Atobe, Hiraku
2020.
On the non-vanishing of theta liftings of tempered representations of U(p,q).
Advances in Mathematics,
Vol. 363,
Issue. ,
p.
106984.
Merino, Allan
2022.
Characters of irreducible unitary representations of 𝑈(𝑛,𝑛+1) via double lifting from 𝑈(1).
Representation Theory of the American Mathematical Society,
Vol. 26,
Issue. 10,
p.
325.