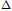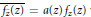In this article we characterize the univalent harmonic mappings from the exterior of the unit disk, $\Delta $, onto a simply connected domain $\Omega $ containing infinity and which are solutions of the system of elliptic partial differential equations $\overline{{{f}_{{\bar{z}}}}\left( Z \right)}=a\left( z \right){{f}_{z}}\left( z \right)$ where the second dilatation function 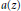$a\left( z \right)$ is a finite Blaschke product. At the end of this article, we apply our results to nonparametric minimal surfaces having the property that the image of its Gauss map is the upper half-sphere covered once or twice.