No CrossRef data available.
Article contents
Existence, Unicite et Multiplicite de Solutions Periodiques D'Equations Differentielles de Duffing Non-Lineaires Avec Dissipation
Published online by Cambridge University Press: 20 November 2018
Extract
Nous nous intéressons à l'existence, l'unicité et la multiplicité de solutions de l'équation différentielle forcée:
1.1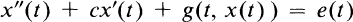
vérifiant les conditions périodiques
1.2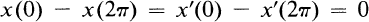
où e ∊ L1(0, 2π), c ∊ R, c arbitraire, g:[0, 2π] × R → R satisfait les conditions de Carathéodory i.e.,g(·, x) est measurable pour tout x ∊ R, g(t, ·) est continu pour presque tout t ∊ [0, 2π].
Le problème (1.1)-(1.2) a été étudié par plusieurs auteurs. Nous mentionerons les travaux [22], [18], [21], [20], [19], [7], [29], [25] et la bibliographie contenue dans ces articles.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1987


