Published online by Cambridge University Press: 20 November 2018
On se donne un intervalle ouvert non vide 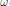 $\omega $ de
$\omega $ de  $\mathbb{R}$, un ouvert connexe non vide
$\mathbb{R}$, un ouvert connexe non vide  $\Omega $ de
$\Omega $ de 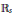 ${{\mathbb{R}}_{5}}$ et unpolynôme unitaire
${{\mathbb{R}}_{5}}$ et unpolynôme unitaire
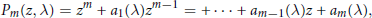 $${{P}_{m}}\left( z,\text{ }\!\!\lambda\!\!\text{ } \right)={{z}^{m}}+{{a}_{1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right){{z}^{m-1}}=+\cdot \cdot \cdot +{{a}_{m-1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)z+{{a}_{m}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)$$
$${{P}_{m}}\left( z,\text{ }\!\!\lambda\!\!\text{ } \right)={{z}^{m}}+{{a}_{1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right){{z}^{m-1}}=+\cdot \cdot \cdot +{{a}_{m-1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)z+{{a}_{m}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)$$
de degré 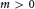 $m>0$, dépendant du paramètre
$m>0$, dépendant du paramètre 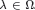 $\lambda \in \Omega $. Un tel polynôme est dit
$\lambda \in \Omega $. Un tel polynôme est dit 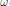 $\omega $-hyperbolique si, pour tout
$\omega $-hyperbolique si, pour tout 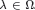 $\lambda \in \Omega $, ses racines sont réelles et appartiennent à
$\lambda \in \Omega $, ses racines sont réelles et appartiennent à 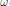 $\omega $.
$\omega $.
On suppose que les fonctions 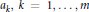 ${{a}_{k}},k=1,\cdot \cdot \cdot ,m$, appartiennent à une classe ultradifférentiable
${{a}_{k}},k=1,\cdot \cdot \cdot ,m$, appartiennent à une classe ultradifférentiable 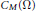 ${{C}_{M}}\left( \Omega \right)$. On s‘intéresse au problème suivant. Soit
${{C}_{M}}\left( \Omega \right)$. On s‘intéresse au problème suivant. Soit  $f$ appartient à
$f$ appartient à 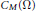 ${{C}_{M}}\left( \Omega \right)$, existe-t-il des fonctions
${{C}_{M}}\left( \Omega \right)$, existe-t-il des fonctions 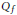 ${{Q}_{f}}$ et
${{Q}_{f}}$ et 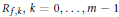 ${{R}_{f,k}},k=0,\cdot \cdot \cdot ,m-1$, appartenant respectivement à
${{R}_{f,k}},k=0,\cdot \cdot \cdot ,m-1$, appartenant respectivement à  ${{C}_{M}}\left( \omega \,\times \,\Omega \right)$ et à
${{C}_{M}}\left( \omega \,\times \,\Omega \right)$ et à 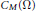 ${{C}_{M}}\left( \Omega \right)$, telles que l’on ait, pour
${{C}_{M}}\left( \Omega \right)$, telles que l’on ait, pour 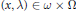 $\left( x,\,\lambda \right)\,\in \,\omega \,\times \,\Omega$,
$\left( x,\,\lambda \right)\,\in \,\omega \,\times \,\Omega$,
 $$f\left( x \right)={{P}_{m}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right){{Q}_{f}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right)+\sum\limits_{k=0}^{m-1}{{{x}^{k}}{{R}_{f,k}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)?}$$
$$f\left( x \right)={{P}_{m}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right){{Q}_{f}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right)+\sum\limits_{k=0}^{m-1}{{{x}^{k}}{{R}_{f,k}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)?}$$
On donne ici une réponse positive dès que le polynôme est 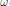 $\omega$-hyperbolique, que la class untradifférentiable soit quasi-analytique ou non ; on obtient alors, des exemples d’idéaux fermés dans
$\omega$-hyperbolique, que la class untradifférentiable soit quasi-analytique ou non ; on obtient alors, des exemples d’idéaux fermés dans 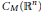 ${{C}_{M}}\left( {{\mathbb{R}}^{n}} \right)$
. On complète ce travail par une généralisation d’un résultat de C. L. Childress dans le cadre quasi-analytique et quelques remarques.
${{C}_{M}}\left( {{\mathbb{R}}^{n}} \right)$
. On complète ce travail par une généralisation d’un résultat de C. L. Childress dans le cadre quasi-analytique et quelques remarques.
Let 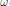 $\omega $ be an open interval in
$\omega $ be an open interval in  $\mathbb{R}$
$\mathbb{R}$ $\Omega $, an open connected set in
$\Omega $, an open connected set in 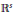 ${{\mathbb{R}}^{s}}$
and
${{\mathbb{R}}^{s}}$
and 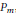 ${{P}_{m}}$
, a monic polynomial
${{P}_{m}}$
, a monic polynomial
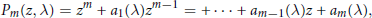 $${{P}_{m}}\left( z,\text{ }\!\!\lambda\!\!\text{ } \right)={{z}^{m}}+{{a}_{1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right){{z}^{m-1}}=+\cdot \cdot \cdot +{{a}_{m-1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)z+{{a}_{m}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)$$
$${{P}_{m}}\left( z,\text{ }\!\!\lambda\!\!\text{ } \right)={{z}^{m}}+{{a}_{1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right){{z}^{m-1}}=+\cdot \cdot \cdot +{{a}_{m-1}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)z+{{a}_{m}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)$$
of degree 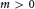 $m>0$, depending on
$m>0$, depending on 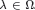 $\lambda \in \Omega $. Such a polynomial is said to be
$\lambda \in \Omega $. Such a polynomial is said to be 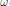 $\omega $-hyperbolic if, for any
$\omega $-hyperbolic if, for any 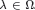 $\lambda \in \Omega $, its roots are real and contained in
$\lambda \in \Omega $, its roots are real and contained in 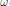 $\omega$.
$\omega$.
Let us suppose that 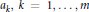 ${{a}_{k}},k=1,\cdot \cdot \cdot ,m$, belong to an ultradifferentiable class
${{a}_{k}},k=1,\cdot \cdot \cdot ,m$, belong to an ultradifferentiable class 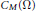 ${{C}_{M}}\left( \Omega \right)$. We deal with the following problem. Given
${{C}_{M}}\left( \Omega \right)$. We deal with the following problem. Given  $f$ belonging to
$f$ belonging to 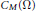 ${{C}_{M}}\left( \Omega \right)$, do there exist functions
${{C}_{M}}\left( \Omega \right)$, do there exist functions 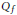 ${{Q}_{f}}$ and
${{Q}_{f}}$ and 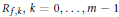 ${{R}_{f,k}},k=0,\cdot \cdot \cdot ,m-1$ belonging respectively to
${{R}_{f,k}},k=0,\cdot \cdot \cdot ,m-1$ belonging respectively to  ${{C}_{M}}\left( \omega \,\times \,\Omega \right)$ and to
${{C}_{M}}\left( \omega \,\times \,\Omega \right)$ and to 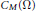 ${{C}_{M}}\left( \Omega \right)$, such that we have, for
${{C}_{M}}\left( \Omega \right)$, such that we have, for 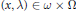 $\left( x,\,\lambda \right)\,\in \,\omega \,\times \,\Omega$,
$\left( x,\,\lambda \right)\,\in \,\omega \,\times \,\Omega$,
 $$f\left( x \right)={{P}_{m}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right){{Q}_{f}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right)+\sum\limits_{k=0}^{m-1}{{{x}^{k}}{{R}_{f,k}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)?}$$
$$f\left( x \right)={{P}_{m}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right){{Q}_{f}}\left( x,\text{ }\!\!\lambda\!\!\text{ } \right)+\sum\limits_{k=0}^{m-1}{{{x}^{k}}{{R}_{f,k}}\left( \text{ }\!\!\lambda\!\!\text{ } \right)?}$$
We give here a positive answer as soon as the polynomial is 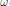 $\omega $-hyperbolic whether the ultradifferentiable class is quasi-analytic or not; we then get examples of closed ideals in
$\omega $-hyperbolic whether the ultradifferentiable class is quasi-analytic or not; we then get examples of closed ideals in 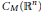 ${{C}_{M}}\left( {{\mathbb{R}}^{n}} \right)$. We complete this work with a generalization of a result of C. L. Childress in the quasi-analytic case and some remarks.
${{C}_{M}}\left( {{\mathbb{R}}^{n}} \right)$. We complete this work with a generalization of a result of C. L. Childress in the quasi-analytic case and some remarks.