Published online by Cambridge University Press: 05 April 2023
Using probabilistic tools, we prove that any weak* continuous semigroup 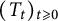 $(T_t)_{t \geqslant 0}$ of self-adjoint unital completely positive measurable Schur multipliers acting on the space
$(T_t)_{t \geqslant 0}$ of self-adjoint unital completely positive measurable Schur multipliers acting on the space  $\mathrm {B}({\mathrm {L}}^2(X))$ of bounded operators on the Hilbert space
$\mathrm {B}({\mathrm {L}}^2(X))$ of bounded operators on the Hilbert space 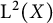 ${\mathrm {L}}^2(X)$, where X is a suitable measure space, can be dilated by a weak* continuous group of Markov
${\mathrm {L}}^2(X)$, where X is a suitable measure space, can be dilated by a weak* continuous group of Markov  $*$-automorphisms on a bigger von Neumann algebra. We also construct a Markov dilation of these semigroups. Our results imply the boundedness of the McIntosh’s
$*$-automorphisms on a bigger von Neumann algebra. We also construct a Markov dilation of these semigroups. Our results imply the boundedness of the McIntosh’s  ${\mathrm {H}}^\infty $ functional calculus of the generators of these semigroups on the associated Schatten spaces and some interpolation results connected to
${\mathrm {H}}^\infty $ functional calculus of the generators of these semigroups on the associated Schatten spaces and some interpolation results connected to  ${\mathrm {BMO}}$-spaces. We also give an answer to a question of Steen, Todorov, and Turowska on completely positive continuous Schur multipliers.
${\mathrm {BMO}}$-spaces. We also give an answer to a question of Steen, Todorov, and Turowska on completely positive continuous Schur multipliers.
The author acknowledges support by the grant ANR-18-CE40-0021 (project HASCON) of the French National Research Agency ANR.