Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ryff, John V
1970.
Measure preserving transformations and rearrangements.
Journal of Mathematical Analysis and Applications,
Vol. 31,
Issue. 2,
p.
449.
Barnes, David C
1972.
Positivity conditions for quadratic forms.
Journal of Mathematical Analysis and Applications,
Vol. 37,
Issue. 3,
p.
607.
1979.
Inequalities - Theory of Majorization and Its Applications.
Vol. 143,
Issue. ,
p.
531.
Barnes, David C.
and
Gilbert, R. P.
1982.
Rearrangements of functions and lower bounds for eigenvalues of differential equations.
Applicable Analysis,
Vol. 13,
Issue. 4,
p.
237.
Barnes, David C.
1983.
Buckling of columns and rearrangements of functions.
Quarterly of Applied Mathematics,
Vol. 41,
Issue. 2,
p.
169.
1988.
Interpolation of Operators.
Vol. 129,
Issue. ,
p.
445.
Mitrinović, D. S.
Pečarić, J. E.
and
Fink, A. M.
1991.
Inequalities Involving Functions and Their Integrals and Derivatives.
p.
285.
Kolyada, V. I.
1992.
Progress in Approximation Theory.
Vol. 19,
Issue. ,
p.
333.
Cianchi, Andrea
2000.
Second-order derivatives and rearrangements.
Duke Mathematical Journal,
Vol. 105,
Issue. 3,
Bhat, M. Ashraf
and
Kosuru, G. Sankara Raju
2022.
On continuity of distribution function and decreasing rearrangement.
Model Assisted Statistics and Applications,
Vol. 17,
Issue. 1,
p.
9.
Bruni, Vittoria
Monteverde, Giuseppina
and
Vitulano, Domenico
2022.
An Entropy-Based Speed Up For Hyperspectral Data Classification Via CNN.
p.
1.
Bruni, Vittoria
Marconi, Silvia
Monteverde, Giuseppina
and
Vitulano, Domenico
2023.
Radon transform of image monotonic rearrangements as feature for noise sensor signature.
Applied Mathematics and Computation,
Vol. 457,
Issue. ,
p.
128173.


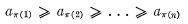
 . Thus the terms of the rearranged sequence
. Thus the terms of the rearranged sequence 