Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T00:24:53.748Z Has data issue: false hasContentIssue false
  • English
  • Français

A Decomposition Theorem for m-Convex Sets in Rd

Published online by Cambridge University Press:  20 November 2018

Marilyn Breen
Marilyn Breen*
Affiliation:
University of Oklahoma, Norman, Oklahoma
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let S be a subset of some linear topological space. The set S is said to be m-convex, m ≧ 2, if and only if for every m-member subset of S, at least one of the 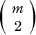line segments determined by these points lies in S. A point x in S is said to be a point of local convexity of S if and only if there is some neighborhood N of x such that if y, z Є N ⌒ S, then [y, z] ⊆ S. If S fails to be locally convex at some point a in S, then q is called a point of local nonconvexity (lnc point) of S.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 28 , Issue 5 , 01 October 1976 , pp. 1051 - 1057
Copyright
Copyright © Canadian Mathematical Society 1976

References

1. Breen, Marilyn, An n -\- 1 member decomposition for sets whose Inc points form n convex sets, Can. J. Math. 27 (1975), 1378–1383.Google Scholar
2. Breen, Marilyn and Kay, David C., General decomposition theorems for m-convex sets in the plane, to appear, Israel J. Math.Google Scholar
3. Lawrence, J. F., Hare, W. R. and Kenelly, John W., Finite unions of convex sets, Proc. Amer. Math. Soc. 34 (1972), 225–228.Google Scholar
4. Stamey, W. L. and Marr, J. M., Unions of two convex sets, Can. J. Math. 15 (1963), 152–156.Google Scholar
5. Valentine, F. A., Convex sets (McGraw-Hill B∞k Co., Inc., New York, 1964).Google Scholar
6. Valentine, F. A., Local convexity and Ln sets, Proc. Amer. Math. Soc. 16 (1965), 1305–1310.Google Scholar
7. Valentine, F. A., A three point convexity property, Pacific J. Math. 7 (1957), 1227–1235.Google Scholar