Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T15:36:02.547Z Has data issue: false hasContentIssue false
  • English
  • Français

A+∞ Condition

Published online by Cambridge University Press:  20 November 2018

F. J. Martín-Reyes ,
L. Pick  and
A. De La Torre
F. J. Martín-Reyes*
Affiliation:
Andlisis Matemdtico Facultad de Ciencias Universidad de Malaga 29071 Malaga Spain, e-mail: MARTIN-REYES@CCUMA UMA ES
L. Pick*
Affiliation:
Mathematical Institute of the Czechoslovak Academy of Sciencesžitna 25 115 67 Prahal, Czechoslovakia, e-mail: [email protected]
A. De La Torre*
Affiliation:
Analisis Matemdtico Facultad de Ciencias Universidad de Malaga 29071 Malaga, Spain, e-mail:[email protected]
*
Current address: School of Mathematics University of Wales College of Cardiff Senghennydd Road Cardiff CF2 4AG United Kingdom, e-mail: PICKL@TAFF CARDIFF AC UK
Current address: School of Mathematics University of Wales College of Cardiff Senghennydd Road Cardiff CF2 4AG United Kingdom, e-mail: PICKL@TAFF CARDIFF AC UK
Current address: School of Mathematics University of Wales College of Cardiff Senghennydd Road Cardiff CF2 4AG United Kingdom, e-mail: PICKL@TAFF CARDIFF AC UK
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

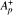
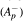The good weights for the one-sided Hardy-Littlewood operators have been characterized by conditions . In this paper we introduce a new condition 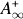which is analogous to A∞. We show several characterizations of 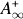. For example, we prove that the class of 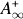weights is the union of 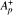classes. We also give a new characterization of 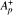weights. Finally, as an application of 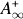condition, we characterize the weights for one-sided fractional integrals and one-sided fractional maximal operators.

Keywords

42B25one-sided Hardy-Littlewood maximal operatorsweighted inequalitiesweightsone-sided fractional operatorsone-sided fractional maximal operatorsRiemann-Liouville fractional integral operator
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 45 , Issue 6 , 01 December 1993 , pp. 1231 - 1244
Copyright
Copyright © Canadian Mathematical Society 1993

Footnotes

This research has been partially supported by D.G.I.C.Y.T. grant (PB88-0324) and Junta de Andalucia.

References

[AS] Andersen, K.F. and Sawyer, E.T., Weighted norm inequalities for the Riemann-Liouville and Weyl fractional integral operators, Trans. Amer. Math. Soc. 308(1988), 547–557.Google Scholar
[BK] Bagby, R.J. andKurtz, D.S., A rearranged good X-inequality, Trans. Amer. Math. Soc. 293(1986), 71–81.Google Scholar
[CF] Coifman, R.R. and Fefferman, C., Weighted norm inequalities for maximal functions and singular integrals, StudiaMath. 51(1974), 241–250.Google Scholar
[GR] Garcia-Cuerva, J. and de Francia, J.L. Rubio, Weighted norm inequalities and related topics, North- Holland, 1985.Google Scholar
[KT] Kerman, R.A. and Torchinsky, A., Integral inequalities with weights for the Hardy maximal function, Studia Math. 71(1982), 277–284.Google Scholar
[K] Kurtz, D.S., Better good X-inequalities, Miniconference on Harmonic Analysis and Operator Algebras Canberra 1987, Proc. Centre Math. Anal. Austral. Nat. Univ. 15, (1987), 118–130.Google Scholar
[M] Martin, F.J.-Reyes, New proofs of weighted inequalities for the one sided Hardy-Littlewood maximal functions, Proc. Amer. Math. Soc. 117(1993), 691–698.Google Scholar
[MOT] Martin, F.J.-Reyes, Salvador, P.Ortega and de la Torre, A. , Weighted inequalities for one-sided maximal functions, Trans. Amer. Math. Soc. 319-2(1990), 517–534.Google Scholar
[MT] Martin-Reyes, F.J. and de la Torre, A., Two weight norm inequalities for fractional one-sided maximal operators, Proc. Amer. Math. Soc. 117(1993), 483–489.Google Scholar
[MW] Muckenhouptand, B. Wheeden, R.L., Weighted norm inequalities for fractional integrals, Trans. Amer. Math. Soc. 192(1974), 261–274.Google Scholar
[Ol] Ortega, P., Weighted inequalities for one sided maximal functions in Orlicz spaces, Studia Math, to appear.Google Scholar
[O2] Ortega, P., Pesos para operadores maximales y teoremas ergodicos en espacios Lp, Lp q y de Orlicz, Doctoral thesis, Universidad de Malaga, 1991.Google Scholar
[S] Sawyer, E., Weighted inequalities for the one sided Hardy-Litlewoodmaximalfunctions, Trans. Amer. Math. Soc. 297(1986), 53–61.Google Scholar
[St] Stein, E.M., Singular integrals and differentiability properties of functions, Princeton Univ. Press, Princeton N.J., 1970.Google Scholar