Let 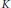$K$ be a real quadratic field, and $p$ a rational prime which is inert in 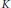$K$. Let 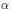$\alpha $ be a modular unit on 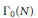${{\Gamma }_{0}}(N)$. In an earlier joint article with Henri Darmon, we presented the definition of an element 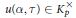$u\left( \alpha ,\,\text{ }\!\!\tau\!\!\text{ } \right)\,\in \,K_{P}^{\times }$ attached to 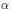$\alpha $ and each 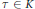$\tau \,\in \,K$. We conjectured that the $p$-adic number 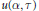$u(\alpha ,\,\tau )$ lies in a specific ring class extension of 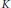$K$ depending on 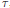$\tau $, and proposed a “Shimura reciprocity law” describing the permutation action of Galois on the set of 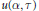$u(\alpha ,\,\tau )$. This article provides computational evidence for these conjectures. We present an efficient algorithm for computing 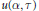$u(\alpha ,\,\tau )$, and implement this algorithm with the modular unit 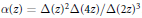$\alpha (z)\,=\,\Delta {{(z)}^{2}}\,\Delta (4z)\,/\,\Delta {{(2z)}^{3}}$. Using 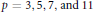$p\,=\,3,\,5,\,7\,and\,11$, and all real quadratic fields $K$ with discriminant 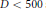$D\,<\,500$ such that 2 splits in 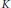$K$ and 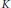$K$ contains no unit of negative norm, we obtain results supporting our conjectures. One of the theoretical results in this paper is that a certain measure used to define 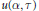$u(\alpha ,\,\tau )$ is shown to be 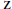$\mathbf{Z}$-valued rather than only 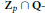${{\mathbf{Z}}_{P}}\,\cap \,\mathbf{Q}-$valued; this is an improvement over our previous result and allows for a precise definition of 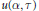$u(\alpha ,\,\tau )$, instead of only up to a root of unity.