Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T00:40:45.702Z Has data issue: false hasContentIssue false
  • English
  • Français

Commutativity Conditions on Rings with Involution

Published online by Cambridge University Press:  20 November 2018

Paola Misso
Paola Misso*
Affiliation:
Università di Palermo, Palermo, Italy
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let R be a ring with involution *. We denote by S, K and Z = Z(R) the symmetric, the skew and the central elements of R respectively.

In [4] Herstein defined the hypercenter T(R) of a ring R as

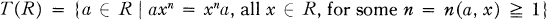
and he proved that in case R is without non-zero nil ideals then T(R) = Z(R).

In this paper we offer a partial extension of this result to rings with involution.

We focus our attention on the following subring of R:

(We shall write H(R) as H whenever there is no confusion as to the ring in question.)

Clearly H contains the central elements of R. Our aim is to show that in a semiprime ring R with involution which is 2 and 3-torsion free, the symmetric elements of H are central.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 34 , Issue 1 , 01 February 1982 , pp. 17 - 22
Copyright
Copyright © Canadian Mathematical Society 1982

References

1. Chacron, M., A commutativity theorem for rings with involution, Can. J. Math. 30 (1978), 1121–1143.Google Scholar
2. Felzenszwalb, B., Rings radical over subrings and some generalization of the notion of center, Univ. of Chicago Thesis (1976).Google Scholar
3. Herstein, I. N., Topics in ring theory (Univ. of Chicago Press, Chicago, 1969).Google Scholar
4. Parmenter, M. M., On the hypercenter of a ring, J. Algebra 36 (1975), 151–157.Google Scholar
5. Parmenter, M. M., Rings with involution (Univ. of Chicago Press, Chicago, 1976).Google Scholar
6. Misso, P., Elementi centrali in un anello primo con involuzione, Atti Accad. Sci. Lett. Arti Palermo (to appear).Google Scholar