Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-24T22:13:07.478Z Has data issue: false hasContentIssue false
  • English
  • Français

Calderón–Zygmund Operators Associated to Ultraspherical Expansions

Published online by Cambridge University Press:  20 November 2018

Dariusz Buraczewski ,
Teresa Martinez  and
José L. Torrea
Dariusz Buraczewski
Affiliation:
Institute of Mathematics, Wroclaw University, Plac Grunwaldzki 2/4, 50-384 Wroclaw, Poland email: [email protected]
Teresa Martinez
Affiliation:
Departamento de Matemáticas, Faculdad de Ciencias, Universidad Autónoma de Madrid, 28049 Madrid, Spain email: [email protected], [email protected]
José L. Torrea
Affiliation:
Departamento de Matemáticas, Faculdad de Ciencias, Universidad Autónoma de Madrid, 28049 Madrid, Spain email: [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define the higher order Riesz transforms and the Littlewood-Paley $g$-function associated to the differential operator 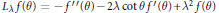${{L}_{\lambda }}f(\theta )\,=\,-{f}''(\theta )-2\lambda \cot \theta {f}'(\theta )+{{\lambda }^{2}}f(\theta )$. We prove that these operators are Calderón–Zygmund operators in the homogeneous type space 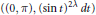$((0,\,\pi ),\,{{(\sin t)}^{2\lambda }}dt)$. Consequently, 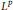${{L}^{p}}$ weighted, ${{H}^{1}}\,-\,{{L}^{1}}$ and 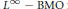${{L}^{\infty }}\,-\,BMO$ inequalities are obtained.

Keywords

42C0542C15ultraspherical polynomialsCalderón–Zygmund operators
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 59 , Issue 6 , 01 December 2007 , pp. 1223 - 1244
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] Buraczewski, D., Martínez, T., Torrea, J. L., and Urban, R., On the Riesz transform associated with ultraspherical expansions. J. Anal. Math. 98(2006), 113–143.Google Scholar
[2] Calderón, A. P., Inequalities for the maximal function relative to a metric. Studia Math. 57(1976), no. 3, 297–306.Google Scholar
[3] Christ, M., Lectures on singular integral operators. CBMS Regional Conference Series in Mathematics 77, American Mathematical Society, Providence, RI, 1990.Google Scholar
[4] Coifman, R. and Weiss, G., Analyse Harmonique non commutative sur certains espaces homogènes. Lecture Notes in Mathematics 242, Springer-Verlag, Berlin, 1971.Google Scholar
[5] Muckenhoupt, B. and Stein, E. M., Classical expansions and their relation to conjugate harmonic functions. Trans. Amer.Math. Soc. 118(1965), 17–92.Google Scholar
[6] Ruiz, F. J., and Torrea, J. L., Vector-valued Calderón–Zygmund theory and Carleson measures on spaces of homogeneous nature. Studia Math. 88(1988), no. 3, 221–243.Google Scholar
[7] Stein, E. M., Topics in Harmonic Analysis Related to the Littlewood–Paley Theory. Annals of Mathematics Studies 63, Princeton University Press, Princeton, NJ, and University of Tokyo Press, Tokyo, 1970.Google Scholar
[8] Szegö, G., Orthogonal Polynomials, American Mathematical Society Colloquium Publications 23, American Mathematical Society, New York, 1939.Google Scholar