Published online by Cambridge University Press: 20 November 2018
A diagonal ind-group is a direct limit of classical affine algebraic groups of growing rank under a class of inclusions that contains the inclusion
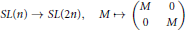 $$SL(n)\,\to \,SL(2n),\,\,M\mapsto \,\left( \begin{matrix}
M & 0 \\
0 & M \\
\end{matrix} \right)$$
$$SL(n)\,\to \,SL(2n),\,\,M\mapsto \,\left( \begin{matrix}
M & 0 \\
0 & M \\
\end{matrix} \right)$$
as a typical special case. If 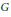 $G$ is a diagonal ind-group and
$G$ is a diagonal ind-group and 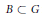 $B\,\subset \,G$ is a Borel ind-subgroup, we consider the ind-variety
$B\,\subset \,G$ is a Borel ind-subgroup, we consider the ind-variety 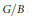 $G/B$ and compute the cohomology
$G/B$ and compute the cohomology  ${{H}^{\ell }}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ of any
${{H}^{\ell }}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ of any 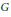 $G$-equivariant line bundle
$G$-equivariant line bundle 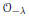 ${{\mathcal{O}}_{-\lambda }}$ on
${{\mathcal{O}}_{-\lambda }}$ on 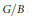 $G/B$. It has been known that, for a generic
$G/B$. It has been known that, for a generic 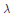 $\lambda $, all cohomology groups of
$\lambda $, all cohomology groups of 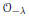 ${{\mathcal{O}}_{-\lambda }}$ vanish, and that a non-generic equivariant line bundle
${{\mathcal{O}}_{-\lambda }}$ vanish, and that a non-generic equivariant line bundle 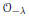 ${{\mathcal{O}}_{-\lambda }}$ has at most one nonzero cohomology group. The new result of this paper is a precise description of when
${{\mathcal{O}}_{-\lambda }}$ has at most one nonzero cohomology group. The new result of this paper is a precise description of when 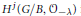 ${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is nonzero and the proof of the fact that, whenever nonzero,
${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is nonzero and the proof of the fact that, whenever nonzero, 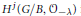 ${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is a
${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is a 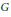 $G$-module dual to a highest weight module. The main difficulty is in defining an appropriate analog
$G$-module dual to a highest weight module. The main difficulty is in defining an appropriate analog  ${{W}_{B}}$ of the Weyl group, so that the action of
${{W}_{B}}$ of the Weyl group, so that the action of  ${{W}_{B}}$ on weights of
${{W}_{B}}$ on weights of 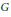 $G$ is compatible with the analog of the Demazure “action” of the Weyl group on the cohomology of line bundles. The highest weight corresponding to
$G$ is compatible with the analog of the Demazure “action” of the Weyl group on the cohomology of line bundles. The highest weight corresponding to 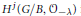 ${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is then computed by a procedure similar to that in the classical Bott–Borel–Weil theorem.
${{H}^{j}}(G/B,\,{{\mathcal{O}}_{-\lambda }})$ is then computed by a procedure similar to that in the classical Bott–Borel–Weil theorem.