Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-23T18:33:39.078Z Has data issue: false hasContentIssue false
  • English
  • Français

Best Approximation in Riemannian Geodesic Submanifolds of Positive Definite Matrices

Published online by Cambridge University Press:  20 November 2018

Yongdo Lim
Yongdo Lim*
Affiliation:
Department of Mathematics, Kyungpook National University, Taegu 702-701, Korea e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We explicitly describe the best approximation in geodesic submanifolds of positive definite matrices obtained from involutive congruence transformations on the Cartan-Hadamard manifold 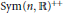$\text{Sym(}n\text{,}\,\mathbb{R}{{\text{)}}^{++}}$ of positive definite matrices. An explicit calculation for the minimal distance function from the geodesic submanifold 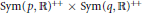$\text{Sym(}p\text{,}\,\mathbb{R}{{\text{)}}^{++}}\,\times \,\text{Sym(}q\text{,}\,\mathbb{R}{{\text{)}}^{++}}$ block diagonally embedded in 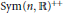$\text{Sym(}n\text{,}\,\mathbb{R}{{\text{)}}^{++}}$ is given in terms of metric and spectral geometric means, Cayley transform, and Schur complements of positive definite matrices when 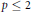$p\,\le \,2$ or 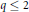$q\,\le \,2$.

Keywords

15A4849R5015A1853C3Matrix approximationpositive definite matrixgeodesic submanifoldCartan-Hadamard manifoldbest approximationminimal distance functionglobal tubular neighborhood theoremSchur complementmetric and spectral geometric meanCayley transform
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 56 , Issue 4 , 01 August 2004 , pp. 776 - 793
Copyright
Copyright © Canadian Mathematical Society 2004

References

[1] Ballmann, W., Lectures on spaces of nonpositive curvature. Birkhäuser, Berlin, 1995.Google Scholar
[2] Borwein, J. M. and Lewis, A. S., Convex analysis and nonlinear optimization. CMS Books in Mathematics, Springer-Verlag, New York, 2000.Google Scholar
[3] Fiedler, M. and Pt ák, V., A new positive definite geometric mean of two positive definite matrices. Linear Algebra Appl, 251(1997), 1–20.Google Scholar
[4] Horn, R. and Johnson, C., Matrix analysis. Cambridge University Press, Cambridge, 1985.Google Scholar
[5] Kubo, F. and Ando, T., Means of positive linear operators. Math. Ann. 246(1980), 205–224.Google Scholar
[6] Lang, S., Fundamentals of differential geometry. Graduate Texts in Mathematics 191, Springer-Verlag, New York, 1999.Google Scholar
[7] Lawson, J. D. and Lim, Y., The geometric mean, matrices, metrics, and more. Amer.Math. Monthly 108(2001), 797–812.Google Scholar
[8] Lewis, A. S., Group invariance and convex matrix analysis. SIAM J. Matrix Anal. Appl 17(1996), 927–949.Google Scholar
[9] Lewis, A. S., Nonsmooth analysis of eigenvalues. Math. Program. 84(1999), 1–24.Google Scholar
[10] Ohara, A., Suda, N. and Amari, S., Dualistic differential geometry of positive definite matrices and its applications to related problems. Linear Algebra Appl. 247(1996), 31–53.Google Scholar
[11] Ohara, A., Information geometric analysis of an interior-point method for semidefinite programming. Proceedings of Geometry in Present Day Science. World Scientific, 1999, pp. 49–74.Google Scholar