Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Goldenberg, H.
1962.
The Evaluation of Inverse Laplace Transforms without the Aid of Contour Integration.
SIAM Review,
Vol. 4,
Issue. 2,
p.
94.
Lew, John S.
1973.
Asymptotic inversion of Laplace transforms: a class of counterexamples.
Proceedings of the American Mathematical Society,
Vol. 39,
Issue. 2,
p.
329.
Handelsman, Richard A.
and
Lew, John S.
1974.
Asymptotic Expansion of Laplace Convolutions for Large Argument and Tail Densities for Certain Sums of Random Variables.
SIAM Journal on Mathematical Analysis,
Vol. 5,
Issue. 3,
p.
425.
1974.
Asymptotics and Special Functions.
p.
548.
Gabutti, Bruno
and
Lepora, Paolo
1987.
A novel approach for the determination of asymptotic expansions of certain oscillatory integrals.
Journal of Computational and Applied Mathematics,
Vol. 19,
Issue. 2,
p.
189.
Venturelli, Davide
and
Gambassi, Andrea
2023.
Memory-induced oscillations of a driven particle in a dissipative correlated medium.
New Journal of Physics,
Vol. 25,
Issue. 9,
p.
093025.
Kalz, Erik
Vuijk, Hidde Derk
Sommer, Jens-Uwe
Metzler, Ralf
and
Sharma, Abhinav
2024.
Oscillatory Force Autocorrelations in Equilibrium Odd-Diffusive Systems.
Physical Review Letters,
Vol. 132,
Issue. 5,
Langer, Amelie
Sharma, Abhinav
Metzler, Ralf
and
Kalz, Erik
2024.
Dance of odd-diffusive particles: A Fourier approach.
Physical Review Research,
Vol. 6,
Issue. 4,
Poh, Yong Rui
and
Yuen-Zhou, Joel
2025.
Stirring Effects on AC-Induced Chemoselectivity in Reversible Electrochemical Reactions.
The Journal of Physical Chemistry C,
Vol. 129,
Issue. 7,
p.
3561.
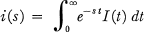
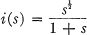 .
.