Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-20T06:34:04.294Z Has data issue: false hasContentIssue false
  • English
  • Français

Ascent Properties of Auslander Categories

Published online by Cambridge University Press:  20 November 2018

Lars Winther Christensen  and
Henrik Holm
Lars Winther Christensen
Affiliation:
Department of Mathematics, University of Britich Columbia, Vancouver, BC, V6T 1Z2,[email protected]
Henrik Holm
Affiliation:
Department of Mathematics, Middlesex College, University of Western Ontario, London, ON, N6A 5B7, [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $R$ be a homomorphic image of a Gorenstein local ring. Recent work has shown that there is a bridge between Auslander categories and modules of finite Gorenstein homological dimensions over $R$.

We use Gorenstein dimensions to prove new results about Auslander categories and vice versa. For example, we establish base change relations between the Auslander categories of the source and target rings of a homomorphism 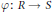$\varphi :\,R\,\to \,S$ of finite flat dimension.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 61 , Issue 1 , 01 February 2009 , pp. 76 - 108
Copyright
Copyright © Canadian Mathematical Society 2009

References

[1] Alonso Tarrìo, L., Jeremìas López, A., and Lipman, J., Local homology and cohomology on schemes. Ann. Sci. ℓcole Norm. Sup. (4) 30 (1997), no. 1, 1–39.Google Scholar
[2] Apassov, D., Homological dimensions over differential graded rings. Complexes and Differential Graded Modules. Ph.D. Thesis, Lund University, 1999, pp. 25–39.Google Scholar
[3] Auslander, M., Anneaux de Gorenstein, et torsion en algèbre commutative. Secrétariat mathématique, Paris, 1967.Google Scholar
[4] Auslander, M. and Bridger, M., Stable module theory. Memoirs of the American Mathematical Society 94, American Mathematical Society, Providence, RI, 1969.Google Scholar
[5] Avramov, L. L. and Foxby, H.-B., Gorenstein local homomorphisms. Bull. Amer. Math. Soc. 23 (1990), no. 1, 145–150.Google Scholar
[6] Avramov, L. L. and Foxby, H.-B., Homological dimensions of unbounded complexes. J. Pure Appl. Algebra 71 (1991), no. 2-3, 129–155.Google Scholar
[7] Avramov, L. L. and Foxby, H.-B., Locally Gorenstein homomorphisms. Amer. J. Math. 114 (1992), no. 5, 1007–1047.Google Scholar
[8] Avramov, L. L. and Foxby, H.-B., Ring homomorphisms and finite Gorenstein dimension. Proc. London Math. Soc. (3) 75 (1997), no. 2, 241–270.Google Scholar
[9] Avramov, L. L. and Foxby, H.-B., Cohen-Macaulay properties of ring homomorphisms. Adv. Math. 133 (1998), no. 1, 54–95.Google Scholar
[10] Avramov, L. L., Foxby, H. B., and Herzog, B., Structure of local homomorphisms. J. Algebra 164 (1994), no. 1, 124–145.Google Scholar
[11] Avramov, L. L., Foxby, H. B., and Lescot, J., Bass series of local ring homomorphisms of finite flat dimension. Trans. Amer.Math. Soc. 335 (1993), no. 2, 497–523.Google Scholar
[12] Avramov, L. L., Iyengar, S., and Miller, C., Homology over local homomorphisms. Amer. J. Math. 128 (2006), no. 1, 23–90.Google Scholar
[13] Avramov, L. L. and Martsinkovsky, A., Absolute, relative, and Tate cohomology of modules of finite Gorenstein dimension. Proc. London Math. Soc. (3) 85 (2002), no. 2, 393–440.Google Scholar
[14] Bass, H., Injective dimension in Noetherian rings. Trans. Amer. Math. Soc. 102 (1962), 18–29.Google Scholar
[15] Cartan, H. and Eilenberg, S., Homological algebra. Princeton Landmarks in Mathematics, Princeton University Press, Princeton, NJ, 1999.Google Scholar
[16] Christensen, L.W., Gorenstein dimensions. Lecture Notes in Mathematics 1747, Springer-Verlag, Berlin, 2000.Google Scholar
[17] Christensen, L.W., Semi-dualizing complexes and their Auslander categories. Trans. Amer. Math. Soc. 353 (2001), no. 5, 1839–1883 (electronic).Google Scholar
[18] Christensen, L.W., Foxby, H.-B., and Frankild, A., Restricted homological dimensions and Cohen-Macaulayness. J. Algebra 251 (2002), no. 1, 479–502.Google Scholar
[19] Christensen, L.W., Frankild, A., and Holm, H., On Gorenstein projective, injective and flat dimensions —A functorial description with applications. J. Algebra 302 (2006), no. 1, 231–279.Google Scholar
[20] Eilenberg, S., Homological dimension and syzygies. Ann. of Math. (2) 64 (1956), 328–336.Google Scholar
[21] Enochs, E. E. and Jenda, O. M. G., Gorenstein injective and projective modules. Math. Z. 220 (1995), no. 4, 611–633.Google Scholar
[22] Enochs, E. E. and Jenda, O. M. G., Relative homological algebra. de Gruyter Expositions in Mathematics 30, Walter de Gruyter & Co., Berlin, 2000.Google Scholar
[23] Enochs, E. E., Jenda, O. M. G., and Torrecillas, B., Gorenstein flat modules. Nanjing Daxue Xuebao Shuxue Bannian Kan 10 (1993), no. 1, 1–9.Google Scholar
[24] Enochs, E. E., Jenda, O. M. G., and Xu, J. Z., Foxby duality and Gorenstein injective and projective modules. Trans. Amer. Math. Soc. 348 (1996), no. 8, 3223–3234.Google Scholar
[25] Foxby, H.-B., Isomorphisms between complexes with applications to the homological theory of modules. Math. Scand. 40 (1977), no. 1, 5–19.Google Scholar
[26] Foxby, H.-B. and Iyengar, S., Depth and amplitude for unbounded complexes. In: Commutative Algebra. Contemp. Math. 331, American Mathematical Society, Providence, RI, 2003, pp. 119–137.Google Scholar
[27] Frankild, A., Vanishing of local homology. Math. Z. 244 (2003), no. 3, 615–630.Google Scholar
[28] Goto, S., Vanishing of Exti A(M, A). J. Math. Kyoto Univ. 22 (1982/83), no. 3, 481–484.Google Scholar
[29] Greenlees, J. P. C. and May, J. P., Derived functors of I-adic completion and local homology. J. Algebra 149 (1992), no. 2, 438–453.Google Scholar
[30] Grothendieck, A., ℓléments de géométrie algébrique. IV. ℓtude locale des schémas et des morphismes de schémas IV, Inst. Hautes ℓtudes Sci. Publ. Math. 32, 1967.Google Scholar
[31] Hartshorne, R., Residues and duality. Lecture notes of a seminar on the work of A. Grothendieck, given at Harvard 1963/64. Lecture Notes in Mathematics 20, Springer-Verlag, Berlin, 1966.Google Scholar
[32] Holm, H., Gorenstein homological dimensions. J. Pure Appl. Algebra 189 (2004), no. 1-3, 167–193.Google Scholar
[33] Holm, H. and Jørgensen, P., Cohen-Macaulay homological dimensions. Rend. Semin.Mat. Univ. Padova 117 (2007), 87–112.Google Scholar
[34] Ishikawa, T., On injective modules and flat modules. J. Math. Soc. Japan 17 (1965), 291–296.Google Scholar
[35] Iyengar, S., Depth for complexes, and intersection theorems.Math. Z. 230 (1999), no. 3, 545–567.Google Scholar
[36] Iyengar, S. and Krause, H., Acyclicity versus total acyclicity for complexes over noetherian rings. Doc. Math. 11 (2006), 207–240.Google Scholar
[37] Iyengar, S. and Sather-Wagstaff, S., G-dimension over local homomorphisms. Applications to the Frobenius endomorphism. Illinois J. Math. 48 (2004), no. 1, 241–272.Google Scholar
[38] Jensen, C. U., On the vanishing of lim −→ (i). J. Algebra 15 (1970), 151–166.Google Scholar
[39] Kaplansky, I., Projective modules. Ann. of Math (2) 68 (1958), 372–377.Google Scholar
[40] Khatami, L. and Yassemi, S., Gorenstein injective and Gorenstein flat dimensions under base change. Comm. Algebra 31 (2003), no. 2, 991–1005.Google Scholar
[41] Matsumura, H., Commutative ring theory. Second ed., Cambridge Studies in Advanced Mathematics 8, Cambridge University Press, Cambridge, 1989. Japanese by M. Reid.Google Scholar
[42] Nagata, M., Local ring. Interscience Tracts in Pure and Applied Mathematics 13, Interscience Publishers a division of John Wiley & Sons New York-London, 1962.Google Scholar
[43] Peskine, C. and Szpiro, L., Dimension projective finie et cohomologie locale. Applications à la démonstration de conjectures de Auslander, M., Bass, H. et Grothendieck, A.. Inst. Hautes ℓtudes Sci. Publ. Math. (1973), no. 42, 47–119.Google Scholar
[44] Raynaud, M. and Gruson, L., Critères de platitude et de projectivité. Techniques de “platification” d’un module. Invent. Math. 13 (1971), 1–89.Google Scholar
[45] Weibel, C. A., An introduction to homological algebra. Cambridge Studies in Advanced Mathematics 38, Cambridge University Press, Cambridge, 1994.Google Scholar
[46] Yassemi, S., G-dimension.Math. Scand. 77 (1995), no. 2, 161–174.Google Scholar
[47] Yoshino, Y., Modules of G-dimension zero over local rings with the cube of maximal ideal being zero. In: Commutative Algebra, singularities and computer algebra (Sinaia, 2002), NATO Sci. Ser. II Math. Phys. Chem. 115, Kluwer, Dordrecht, 2003, pp. 255–273.Google Scholar