No CrossRef data available.
Article contents
Analytic Equivalence of Algebroid Curves
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let k be an algebraically closed field and let x1, x2, . . . , xn be indeterminates. Denote by Rn the ring k[[x1, x2, … , xn]] of power series in the xi With coefficients in the field k. Let 
and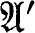 
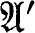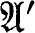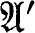be two ideals in this ring. Then and will be said to be analytically equivalent if there is an automorphism T of Rn such that T() = . and will be called analytically equivalent under T.
The above situation can be described geometrically as follows. The ideals and 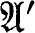can be regarded as defining algebroid varieties V and V' in (x1, x2, … , xn)-space, and these varieties will be said to be analytically equivalent under T.
The automorphism T can be expressed by means of equations of the form :
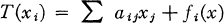
where the determinant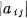 
is not zero and the fi are power series of order not less than two (that is to say, containing terms of degree two or more only).
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1959
References
2.
Chevalley, C., Intersections of algebraic and algebroid varieties, Trans. Amer. Math. Soc, 57 (1945), 1–85.Google Scholar
3.
Cohen, I. S., On the structure and ideal theory of complete local rings, Trans. Amer. Math. Soc, 59 (1946), 54–106.Google Scholar
4.
Samuel, P., L’algébricité de certains points singuliers algébroides, J. de Math, pures et appl., (9), 85 (1956), 1–6.Google Scholar
6.
Wallace, A. H., Algebraic approximation of curves, Can. J. Math., 10 (1958), 242–278.Google Scholar
You have
Access