Real functions

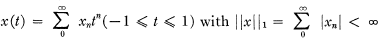
may be identified with elements x = (x0, x1, x2, … ) of the sequence space l1 Since the unit sphere S∞ of l1 is compact under the weak* topology = topology of co-ordinatewise convergence, a countably additive measure on S∞ is induced by a positive linear functional E (integral) on C(S∞), the weak* continuous real-valued functions on S∞. There exists a natural integral over S∞ reducing to

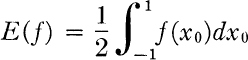
when f is a function of x0 alone. The partial sums Sn = Sn(x) of the power series for x(t) then form a martingale and zero-or-one phenomena appear. In particular, if R(x) is the radius of convergence of the series and e is the base of the natural logarithms, it turns out that R(x) = e for almost all x in S∞. Applications of the integral to the theory of numerical integration, the original motivation, will appear in a later paper.