Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-05T11:55:58.232Z Has data issue: false hasContentIssue false
  • English
  • Français

Almost Convergence, Summability And Ergodicity

Published online by Cambridge University Press:  20 November 2018

J. Peter Duran
J. Peter Duran*
Affiliation:
University of Puerto Rico, Mayaguez, Puerto Rico
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

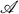The notion of almost convergence introduced by Lorentz [15] has been generalized in several directions (see, for example [1; 8; 11 ; 14; 17]). I t is the purpose of this paper to give a generalization based on the original definition in terms of invariant means. This is effected by replacing the shift transformation by an "ergodic" semigroup of positive regular matrices in the definition of invariant mean. The resulting "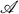- invariant means" give rise to a summability method which we dub 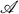-almost convergence.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 26 , Issue 2 , April 1974 , pp. 372 - 387
Copyright
Copyright © Canadian Mathematical Society 1974

References

1. Atalla, R., On the inclusion of a bounded convergence field in the space of almost convergent sequences, Glasgow Math. J. 31 (1972), 82–90.Google Scholar
2. Borwein, D., A logarithmic method of summability, J. London Math. Soc. 38 (1958), 212–220.Google Scholar
3. Chou, C., The multipliers for the space of almost convergent sequences, Illinois J. Math. 16 (1972), 687–694.Google Scholar
4. Chou, C. and Duran, J. P., Multipliers for the space of almost-convergent functions on a semigroup, Proc. Amer. Math. Soc. 39 (1973), 125–128.Google Scholar
5. Day, M. M., Means for the bounded functions and ergodicity of bounded representations of semigroups, Trans. Amer. Math. Soc. 69 (1950), 276–291.Google Scholar
6. Dean, D. and Raimi, R. A., Permutations with comparable sets of invariant means, Duke Math. J. 27 (1960), 467–480.Google Scholar
7. Eberlein, W. F., Abstract ergodic theorems and weak almost periodic functions, Trans. Amer. Math. Soc. 67 (1949), 217–240.Google Scholar
8. Eberlein, W. F., Banach-Hausdorff limits, Proc. Amer. Math. Soc. 1 (1950), 662–665.Google Scholar
9. Eberlein, W. F., On Hölder summability of infinite order, Notices Amer. Math. Soc. 19 (1972), A-164; Abstract #691-46-21.Google Scholar
10. Fuchs, W. H. J., On the “collective Hausdorff method”, Proc. Amer. Math. Soc. 1 (1950), 26–30.Google Scholar
11. Garten, V. and Knopp, K., Ungleichungen zwischen Mittlewerten von Zahlenfolgen und Funktionen, Math. Z. 42 (1937), 365–388.Google Scholar
12. Hardy, G. H., Divergent series (Oxford University Press, Oxford, 1949).Google Scholar
13. Jerison, M., On the set of generalized limits of bounded sequences, Can. J. Math. 9 (1957), 79–89.Google Scholar
14. King, J. P., Almost summable sequences, Proc. Amer. Math. Soc. 17 (1966), 1219–1225.Google Scholar
15. Lorentz, G. G., A contribution to the theory of divergent sequences, Acta Math. 80 (1948), 167–190.Google Scholar
16. Petersen, G. M., Almost convergence and uniformly distributed sequences, Quart. J. Math. Oxford Ser. 2, 7 (1956), 188–191.Google Scholar
17. Raimi, R. A., On Banach's generalized limits, Duke Math. J. 26 (1959), 17–28.Google Scholar
18. Raimi, R. A., Invariant means and invariant matrix methods of summability, Duke Math. J. 33 (1966), 1–12.Google Scholar
19. Schaefer, P., Matrix transformations of almost convergent sequences, Math. Z. 112 (1969), 321–325.Google Scholar