Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-01-08T07:48:06.010Z Has data issue: false hasContentIssue false
  • English
  • Français

Affine isoperimetric inequalities on flag manifolds

Part of: General convexity

Published online by Cambridge University Press:  19 December 2023

Susanna Dann ,
Grigoris Paouris  and
Peter Pivovarov
Susanna Dann*
Affiliation:
Departamento de Matemáticas, Universidad de los Andes, Carrera 1 No. 18A-12, 111711 Bogotá, Colombia
Grigoris Paouris
Affiliation:
Department of Mathematics, Mailstop 3368, Texas A&M University, College Station, TX 77843-3368, United States e-mail: [email protected]
Peter Pivovarov
Affiliation:
Mathematics Department, University of Missouri, Columbia, MO 65211, United States e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Building on work of Furstenberg and Tzkoni, we introduce 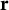$\mathbf {r}$-flag affine quermassintegrals and their dual versions. These quantities generalize affine and dual affine quermassintegrals as averages on flag manifolds (where the Grassmannian can be considered as a special case). We establish affine and linear invariance properties and extend fundamental results to this new setting. In particular, we prove several affine isoperimetric inequalities from convex geometry and their approximate reverse forms. We also introduce functional forms of these quantities and establish corresponding inequalities.

Keywords

Flag manifolds(dual) affine quermassintegrals(approximate reverse) isoperimetric inequalitiesaffine invariance

MSC classification

Primary: 52A22: Random convex sets and integral geometry
Type
Article
Information
Canadian Journal of Mathematics , First View , pp. 1 - 30
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Susanna Dann was supported by the FAPA funds from Vicerrectoría de Investigaciones de la Universidad de los Andes (INV-2019-63-1699). Grigoris Paouris was supported by Simons Foundation Collaboration Grant #527498 and NSF grant DMS-1812240. Peter Pivovarov was supported by NSF grant DMS-1612936.

References

Artstein-Avidan, S., Klartag, B., and Milman, V., The Santaló point of a function, and a functional form of the Santaló inequality . Mathematika 51(2004), nos. 1–2, 33–48.10.1112/S0025579300015497CrossRefGoogle Scholar
Artstein-Avidan, S., Klartag, B., Schütt, C., and Werner, E., Functional affine-isoperimetry and an inverse logarithmic Sobolev inequality . J. Funct. Anal. 262(2012), no. 9, 4181–4204.10.1016/j.jfa.2012.02.014CrossRefGoogle Scholar
Ball, K. M., Logarithmically concave functions and sections of convex sets in ${\mathbb{R}}^n$ . Stud. Math. 88(1988), 69–84.10.4064/sm-88-1-69-84CrossRefGoogle Scholar
Bobkov, S. G., Colesanti, A., and Fragalá, I., Quermassintegrals of quasi-concave functions and generalized Prékopa–Leindler inequalities . Manuscripta Math. 143(2014), nos. 1–2, 131–169.CrossRefGoogle Scholar
Bourgain, J. and Milman, V. D., New volume ratio properties for convex symmetric bodies in ${\mathbb{R}}^n$ . Invent. Math. 88(1987), no. 2, 319–340.CrossRefGoogle Scholar
Brazitikos, S., Giannopoulos, A., Valettas, P., and Vritsiou, B. H., Geometry of isotropic convex bodies, Mathematical Surveys and Monographs, 196, American Mathematical Society, Providence, RI, 2014.Google Scholar
Bürgisser, P. and Lerario, A., Probabilistic Schubert calculus . J. Reine Angew. Math. 760(2020), 1–58.10.1515/crelle-2018-0009CrossRefGoogle Scholar
Busemann, H., Volume in terms of concurrent cross-sections . Pacific J. Math. 3(1953), 1–12.10.2140/pjm.1953.3.1CrossRefGoogle Scholar
Busemann, H. and Straus, E. G., Area and normality . Pacific J. Math. 10(1960), 35–72.10.2140/pjm.1960.10.35CrossRefGoogle Scholar
Cordero-Erausquin, D., Fradelizi, M., Paouris, G., and Pivovarov, P., Volume of the polar of random sets and shadow systems . Math. Ann. 362(2015), nos. 3–4, 1305–1325.10.1007/s00208-014-1156-xCrossRefGoogle Scholar
Dafnis, N. and Paouris, G., Estimates for the affine and dual affine quermassintegrals of convex bodies . Ill. J. Math. 56(2012), no. 4, 1005–1021.Google Scholar
Dann, S., Paouris, G., and Pivovarov, P., Bounding marginal densities via affine isoperimetry . Proc. Lond. Math. Soc. 113(2016), 140–162.10.1112/plms/pdw026CrossRefGoogle Scholar
Figiel, T. and Tomczak-Jaegermann, N., Projections onto Hilbertian subspaces of Banach spaces . Israel J. Math. 33(1979), 155–171.CrossRefGoogle Scholar
Fradelizi, M. and Meyer, M., Some functional forms of Blaschke–Santaló inequality . Math. Z. 256(2007), no. 2, 379–395.10.1007/s00209-006-0078-zCrossRefGoogle Scholar
Furstenberg, H. and Keston, H., Products of random matrices . Ann. Math. Statist. 31(1960), 457–469.10.1214/aoms/1177705909CrossRefGoogle Scholar
Furstenberg, H. and Tzkoni, I., Spherical functions and integral geometry . Israel J. Math. 10(1971), 327–338.CrossRefGoogle Scholar
Gardner, R. J., Geometric tomography, 2nd ed., Cambridge University Press, New York, 2006.Google Scholar
Gardner, R. J., The dual Brunn–Minkowski theory for bounded Borel sets: dual affine quermassintegrals and inequalities . Adv. Math. 216(2007), no. 1, 358–386.10.1016/j.aim.2007.05.018CrossRefGoogle Scholar
Giannopoulos, A., Paouris, G., and Vritsiou, B. H., The isotropic position and the reverse Santaló inequality . Israel J. Math. 203(2014), no. 1, 1–22.10.1007/s11856-012-0173-2CrossRefGoogle Scholar
Grinberg, E. L., Isoperimetric inequalities and identities for k-dimensional cross-sections of convex bodies . Math. Ann. 291(1991), no. 1, 75–86.CrossRefGoogle Scholar
Hanin, B. and Paouris, G., Non-asymptotic results for singular values of Gaussian matrix products . Geom. Funct. Anal. 31(2021), no. 2, 268–324.CrossRefGoogle Scholar
Huang, Y., Lutwak, E., Yang, D., and Zhang, G., Geometric measures in the dual Brunn–Minkowski theory and their associated Minkowski problems . Acta Math. 216(2016), no. 2, 325–388.CrossRefGoogle Scholar
Hug, D., Rataj, J., and Weil, W., Flag representations of mixed volumes and mixed functionals of convex bodies . J. Math. Anal. Appl. 460(2018), no. 2, 745–776.10.1016/j.jmaa.2017.12.039CrossRefGoogle Scholar
Klartag, B., On convex perturbations with a bounded isotropic constant . Geom. Funct. Anal. 16(2006), no. 6, 1274–1290.10.1007/s00039-006-0588-1CrossRefGoogle Scholar
Klartag, B. and Milman, V., Geometry of $\mathit{log}$ -concave functions and measures . Geom. Dedicata. 112(2005), no. 1, 169–182.10.1007/s10711-004-2462-3CrossRefGoogle Scholar
Kuperberg, G., From the Mahler conjecture to gauss linking integrals . Geom. Funct. Anal. 18(2008), no. 3, 870–892.10.1007/s00039-008-0669-4CrossRefGoogle Scholar
Lehec, J., The symmetric property ( $\tau$ ) for the Gaussian measure . Ann. Fac. Sci. Toulouse Math. (6) 17(2008), no. 2, 357–370.CrossRefGoogle Scholar
Lewis, D. R., Ellipsoids defined by Banach ideal norms . Mathematika 26(1979), 18–29.CrossRefGoogle Scholar
Lutwak, E., Dual mixed volumes . Pacific J. Math. 58(1975), no. 2, 531–538.10.2140/pjm.1975.58.531CrossRefGoogle Scholar
Lutwak, E., A general isepiphanic inequality . Proc. Amer. Math. Soc. 90(1984), no. 3, 415–421.CrossRefGoogle Scholar
Lutwak, E., Intersection bodies and dual mixed volumes . Adv. Math. 71(1988), no. 2, 232–261.10.1016/0001-8708(88)90077-1CrossRefGoogle Scholar
Lutwak, E., Inequalities for Hadwiger’s harmonic quermassintegrals . Math. Ann. 280(1988), no. 1, 165–175.10.1007/BF01474188CrossRefGoogle Scholar
Lutwak, E., Extended affine surface area . Adv. Math. 85(1991), no. 1, 39–68.CrossRefGoogle Scholar
Lutwak, E., Selected affine isoperimetric inequalities . In: Handbook of convex geometry, Vols. A and B, North-Holland, Amsterdam, 1993, pp. 151–176.10.1016/B978-0-444-89596-7.50010-9CrossRefGoogle Scholar
Lutwak, E., Yang, D., and Zhang, G., ${L}_p$ affine isoperimetric inequalities . J. Differ. Geom. 56(2000), no. 1, 111–132.10.4310/jdg/1090347527CrossRefGoogle Scholar
Lutwak, E., Yang, D., and Zhang, G., Sharp affine ${L}_p$ -Sobolev inequalities . J. Differ. Geom. 62(2002), 17–38.CrossRefGoogle Scholar
Lutwak, E. and Zhang, G., Blaschke–Santaló inequalities . J. Differ. Geom. 47(1997), no. 1, 1–16.10.4310/jdg/1214460036CrossRefGoogle Scholar
Milman, E. and Yehudayoff, A., Sharp isoperimetric inequalities for affine quermassintegrals . J. Amer. Math. Soc. 36(2023), no. 4, 1061–1101.Google Scholar
Milman, V. and Rotem, L., Mixed integrals and related inequalities . J. Funct. Anal. 264(2013), no. 2, 570–604.10.1016/j.jfa.2012.10.019CrossRefGoogle Scholar
Milman, V. D., Isomorphic symmetrization and geometric inequalities . In: Geometric aspects of functional analysis (1986/87), Lecture Notes in Mathematics, 1317, Springer, Berlin, 1988, pp. 107–131.10.1007/BFb0081738CrossRefGoogle Scholar
Nazarov, F., The Hörmander proof of the Bourgain–Milman theorem . In: Geometric aspects of functional analysis, Lecture Notes in Mathematics, 2050, Springer, Heidelberg, 2012, pp. 335–343.CrossRefGoogle Scholar
Paouris, G. and Pivovarov, P., Small-ball probabilities for the volume of random convex sets . Discrete Comput. Geom. 49(2013), no. 3, 601–646.CrossRefGoogle Scholar
Paouris, G. and Valettas, P., Neighborhoods on the Grassmannian of marginals with bounded isotropic constant . J. Funct. Anal. 267(2014), no. 9, 3427–3443.10.1016/j.jfa.2014.07.004CrossRefGoogle Scholar
Petty, C. M., Isoperimetric problems . In: Proceedings of the conference on convexity and combinatorial geometry (Univ. Oklahoma, Norman, Okla., 1971), Department of Mathematics, The University of Oklahoma, Norman, OH, 1971, pp. 26–41.Google Scholar
Pisier, G., Holomorphic semi-groups and the geometry of Banach spaces . Ann. of Math. 115(1982), 375–392.10.2307/1971396CrossRefGoogle Scholar
Pisier, G., The volume of convex bodies and Banach space geometry, Cambridge Tracts in Mathematics, 94, Cambridge University Press, Cambridge, 1989.Google Scholar
Rogers, C. A. and Shephard, G., The difference body of a convex body . Arch. Math. 8(1957), 220–233.10.1007/BF01899997CrossRefGoogle Scholar
Santaló, L. A., An affine invariant for convex bodies of $n$ -dimensional space . Portugaliae Math. 8(1949), 155–161.Google Scholar
Schneider, R., Convex bodies: the Brunn–Minkowski theory, second expanded ed., Encyclopedia of Mathematics and Its Applications, 151, Cambridge University Press, Cambridge, 2014.Google Scholar
Schneider, R. and Weil, W., Zonoids and related topics . In: Convexity and its applications, Birkhäuser, Basel, 1983, pp. 296–317.CrossRefGoogle Scholar
Schneider, R. and Weil, W., Stochastic and integral geometry, probability and its applications (New York), Springer, Berlin, 2008.Google Scholar
Tomczak-Jaegermann, N., Banach–Mazur distances and finite-dimensional operator ideals, Pitman Monographs and Surveys in Pure and Applied Mathematics, 38, Longman Scientific & Technical, Harlow, 1989.Google Scholar
Zhang, G., The affine Sobolev inequality. J. Differ. Geom. 53(1999), no. 1, 183–202.CrossRefGoogle Scholar