Let an interval I ⊂ ℝ and subsets D0, D1 ⊂ I with D0 ∪ D1 = I and D0 ∩ D1 = Ø be given, as well as functions r0: D0 → I, r1: D1 → I. We investigate the system (S) of two functional equations for an unknown function f: I → [0, 1]: 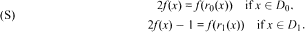We derive conditions for the existence, continuity and monotonicity of a solution. It turns out that the binary expansion of a solution can be computed in a simple recursive way. This recursion is algebraic for, e.g., inverse trigonometric functions, but also for the elliptic integral of the first kind. Moreover, we use (S) to construct two kinds of peculiar functions: surjective functions whose intervals of constancy are residual in I, and strictly increasing functions whose derivative is 0 almost everywhere.