Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-02-07T04:24:02.606Z Has data issue: false hasContentIssue false
  • English
  • Français

On the compositum of orthogonal cyclic fields of the same odd prime degree

Part of: Algebraic number theory: global fields

Published online by Cambridge University Press:  14 July 2020

Cornelius Greither  and
Radan Kučera
Cornelius Greither
Affiliation:
Universität der Bundeswehr München, Neubiberg, Germany e-mail: [email protected]
Radan Kučera*
Affiliation:
Masaryk University, Brno, Czech Republic
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The aim of this paper is to study circular units in the compositum K of t cyclic extensions of 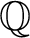${\mathbb {Q}}$ (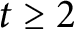 $t\ge 2$ ) of the same odd prime degree $\ell $ . If these fields are pairwise arithmetically orthogonal and the number s of primes ramifying in $K/{\mathbb {Q}}$ is larger than $t,$ then a nontrivial root $\varepsilon $ of the top generator $\eta $ of the group of circular units of K is constructed. This explicit unit 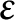$\varepsilon $ is used to define an enlarged group of circular units of K, to show that 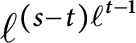$\ell ^{(s-t)\ell ^{t-1}}$ divides the class number of K, and to prove an annihilation statement for the ideal class group of K.

Keywords

Circular (cyclotomic) unitsabsolutely abelian fieldsclass groups

MSC classification

Primary: 11R20: Other abelian and metabelian extensions
Secondary: 11R27: Units and factorization
Type
Article
Information
Canadian Journal of Mathematics , Volume 73 , Issue 6 , December 2021 , pp. 1506 - 1530
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

R. K. was supported by Project 18-11473S of the Czech Science Foundation.

References

Greither, C. and Kučera, R., Annihilators for the class group of a cyclic field of prime power degree . Acta Arith. 112(2004), 177–198. http://dx.doi.org/10.4064/aa112-2-6 CrossRefGoogle Scholar
Greither, C. and Kučera, R., Annihilators for the class group of a cyclic field of prime power degree III . Publ. Math. 86(2015), 401–421. http://dx.doi.org/10.5486/PMD.2015.7029 Google Scholar
Greither, C. and Kučera, R., Linear forms on Sinnott’s module . J. Number Theory 141(2014), 324–342. http://dx.doi.org/10.1016/j.jnt.2014.02.003 CrossRefGoogle Scholar
Greither, C. and Kučera, R., Washington units, semispecial units, and annihilation of class groups. Manuscripta Math. 2020. http://dx.doi.org/10.1007/s00229-020-01241-y CrossRefGoogle Scholar
Herman, J., Annihilators of the class group of a compositum of quadratic fields . Arch. Math. (Brno) 49(2013), 209–222. http://dx.doi.org/10.5817/AM2013-4-209 CrossRefGoogle Scholar
Kučera, R., On the class number of a compositum of real quadratic fields: an approach via circular units . Funct. Approx. Comment. Math. 39(2008), 179–189. http://dx.doi.org/10.7169/facm/1229696569 CrossRefGoogle Scholar
Lettl, G., A note on Thaine’s circular units . J. Number Theory 35(1990), 224–226. http://dx.doi.org/10.1016/0022-314X(90)90115-8 CrossRefGoogle Scholar
Sinnott, W., On the Stickelberger ideal and the circular units of an abelian field . Invent. Math. 62(1980), 181–234. http://dx.doi.org/10.1007/BF01389158 CrossRefGoogle Scholar