Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T07:04:56.788Z Has data issue: false hasContentIssue false
  • English
  • Français

Further inequalities and properties of p-inner parallel bodies

Part of: General convexity

Published online by Cambridge University Press:  09 November 2020

Yingying Lou ,
Dongmeng Xi  and
Zhenbing Zeng
Yingying Lou*
Affiliation:
Department of Mathematics, Shanghai University, Shanghai200444, China e-mail: [email protected]@shu.edu.cn
Dongmeng Xi
Affiliation:
Department of Mathematics, Shanghai University, Shanghai200444, China e-mail: [email protected]@shu.edu.cn
Zhenbing Zeng
Affiliation:
Department of Mathematics, Shanghai University, Shanghai200444, China e-mail: [email protected]@shu.edu.cn
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A. R. Martínez Fernández obtained upper bounds for quermassintegrals of the p-inner parallel bodies: an extension of the classical inner parallel body to the 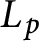$L_p$ -Brunn-Minkowski theory. In this paper, we establish (sharp) upper and lower bounds for quermassintegrals of p-inner parallel bodies. Moreover, the sufficient and necessary conditions of the equality case for the main inequality are obtained, which characterize the so-called tangential bodies.

Keywords

p-Inner parallel body0-extreme normal vectortangential body

MSC classification

Primary: 52A20: Convex sets in $n$ dimensions (including convex hypersurfaces)
Secondary: 52A39: Mixed volumes and related topics 52A40: Inequalities and extremum problems
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 2 , April 2022 , pp. 368 - 380
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

D. X. was supported by the NSFC 11601310. Z. Z. was supported by the NSFC 11471209.

References

Firey, W. J., $p$ -Means of convex bodies . Math. Scand. 10(1962), 17–24. https://doi.org/10.7146/math.scand.a-10510 CrossRefGoogle Scholar
Gardner, R. J., Geometric tomography . 2nd ed., Encyclopedia of Mathematics and its Applications, 58, Cambridge University Press, Cambridge, MA, 2006. http://doi.org/10.1017/CBO9781107341029 CrossRefGoogle Scholar
Hardy, G. H., Littlewood, J. E., and Pólya, G., Inequalities . 2nd ed., Cambridge University Press, Cambridge, MA, 1952.Google Scholar
Cifre, M. A. Hernández, Fernández, A. R. Martínez, and Gómez, E. Saorín, Differentiability properties of the family of $p$ -parallel bodies . Appl. Anal. Discrete Math. 10(2016), no. 1, 186–207. https://doi.org/10.2298/aadm160325005c CrossRefGoogle Scholar
Cifre, M. A. Hernández and Saorín, E., On inner parallel bodies and quermassintegrals . Israel J. Math. 177(2010), 29–47. https://doi.org/10.1007/s11856-010-0037-6 CrossRefGoogle Scholar
Lutwak, E., The Brunn–Minkowski–Firey theory. I. Mixed volumes and the Minkowski problem . J. Differ. Geom. 38(1993), no. 1, 131–150. https://doi.org/10.4310/jdg/1214454097 Google Scholar
Lutwak, E., The Brunn–Minkowski–Firey theory II: Affine and geominimal surface areas . Adv. Math. 118(1996), no. 2, 244–294. https://doi.org/10.1006/aima.1996.0022 CrossRefGoogle Scholar
Fernández, A. R. Martínez, Going further in the ${L}_p$ -Brunn–Minkowski theory: a $p$ -difference of convex bodies. Ph. D. thesis, University of Murcia, 2016. https://doi.org/10.13140/RG.2.2.22938.44482 CrossRefGoogle Scholar
Fernández, A. R. Martínez, Gómez, E. Saorín, and Nicolás, J. Yepes, $p$ -difference: a counterpart of Minkowski difference in the framework of the ${L}_p$ -Brunn–Minkowski theory . Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. RACSAM 110(2016), no. 2, 613–631. https://doi.org/10.1007/s13398-015-0253-3 Google Scholar
Schneider, R., On the Aleksandrov–Fenchel inequality . Ann. N. Y. Acad. Sci. 440(1985), no. 1, 132–141. https://doi.org/10.1111/j.1749-6632.1985.tb14547.x CrossRefGoogle Scholar
Schneider, R., Convex bodies: The Brunn–Minkowski theory . 2nd ed., Encyclopedia of Mathematics and its Applications, 151, Cambridge University Press, Cambridge, MA, 2014.Google Scholar