Article contents
An Application of Spherical Geometry to Hyperkähler Slices
Published online by Cambridge University Press: 24 February 2020
Abstract
This work is concerned with Bielawski’s hyperkähler slices in the cotangent bundles of homogeneous affine varieties. One can associate such a slice with the data of a complex semisimple Lie group 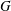 $G$, a reductive subgroup
$G$, a reductive subgroup 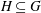 $H\subseteq G$, and a Slodowy slice
$H\subseteq G$, and a Slodowy slice 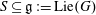 $S\subseteq \mathfrak{g}:=\text{Lie}(G)$, defining it to be the hyperkähler quotient of
$S\subseteq \mathfrak{g}:=\text{Lie}(G)$, defining it to be the hyperkähler quotient of  $T^{\ast }(G/H)\times (G\times S)$ by a maximal compact subgroup of
$T^{\ast }(G/H)\times (G\times S)$ by a maximal compact subgroup of 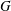 $G$. This hyperkähler slice is empty in some of the most elementary cases (e.g., when
$G$. This hyperkähler slice is empty in some of the most elementary cases (e.g., when 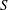 $S$ is regular and
$S$ is regular and 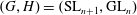 $(G,H)=(\text{SL}_{n+1},\text{GL}_{n})$,
$(G,H)=(\text{SL}_{n+1},\text{GL}_{n})$, 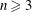 $n\geqslant 3$), prompting us to seek necessary and sufficient conditions for non-emptiness.
$n\geqslant 3$), prompting us to seek necessary and sufficient conditions for non-emptiness.
We give a spherical-geometric characterization of the non-empty hyperkähler slices that arise when 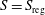 $S=S_{\text{reg}}$ is a regular Slodowy slice, proving that non-emptiness is equivalent to the so-called
$S=S_{\text{reg}}$ is a regular Slodowy slice, proving that non-emptiness is equivalent to the so-called  $\mathfrak{a}$-regularity of
$\mathfrak{a}$-regularity of  $(G,H)$. This
$(G,H)$. This  $\mathfrak{a}$-regularity condition is formulated in several equivalent ways, one being a concrete condition on the rank and complexity of
$\mathfrak{a}$-regularity condition is formulated in several equivalent ways, one being a concrete condition on the rank and complexity of 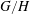 $G/H$. We also provide a classification of the
$G/H$. We also provide a classification of the  $\mathfrak{a}$-regular pairs
$\mathfrak{a}$-regular pairs 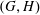 $(G,H)$ in which
$(G,H)$ in which  $H$ is a reductive spherical subgroup. Our arguments make essential use of Knop’s results on moment map images and Losev’s algorithm for computing Cartan spaces.
$H$ is a reductive spherical subgroup. Our arguments make essential use of Knop’s results on moment map images and Losev’s algorithm for computing Cartan spaces.
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
The first author is supported by the Natural Sciences and Engineering Research Council of Canada [PDF–516638–2018].
References
- 2
- Cited by



