Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-28T05:01:27.887Z Has data issue: false hasContentIssue false
  • English
  • Français

SAMPLING STATISTICS AND A SAMPLING SCHEME FOR THE TWOSPOTTED SPIDER MITE, TETRANYCHUS URTICAE (ACARI: TETRANYCHIDAE), ON STRAWBERRIES

Published online by Cambridge University Press:  31 May 2012

D.A. Raworth
D.A. Raworth
Affiliation:
Agriculture Canada Research Station, 6660 N.W. Marine Drive, Vancouver, British Columbia, Canada V6T 1X2
Get access Rights & Permissions [Opens in a new window]

Abstract

A series of samples of the twospotted spider mite, Tetranychus urticae Koch, from 16 experimental populations on “Totem” strawberry, Fragaria × ananassa Duch., were examined to determine the index of dispersion, the variance–mean relationship, the distribution, and the relationship between the mean number of T. urticae/leaflet and the proportion of leaflets without T. urticae. The slope of the variance–mean relationship, 1.64 ± 0.0355(SE), did not differ between sample dates but the intercept decreased significantly (p < 0.01) from 2.56 ± 0.0969 before harvest to 1.76 ± 0.140 during harvest. At 0.0136 T. urticae/leaflet before harvest and 0.0644 T. urticae/leaflet during harvest, the variance equaled the mean, implying that at these densities the data followed the Poisson distribution. Above these densities the data were overdispersed, most samples conforming with the negative binomial distribution but some tending towards greater dispersion than the negative binomial. There was no common k for the negative binomial nor did the data fit the expectation for 1/k that was consistent with the variance–mean relationship. A distribution-free sampling scheme based on the sample mean and the proportion of leaflets without T. urticae (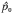) was developed. Tetranychus urticae density can be quickly determined in the field using the naked eye, by iteratively observing leaflets for the presence or absence of T. urticae and referring to a small table that gives both the mean density for a given 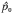and the number of leaflets required to obtain a specified standard error.

Résumé

Une série d’échantillons du tétranyque à deux points, Tetranychus urticae Koch, prélevés de 16 populations expérimentales sur le fraisier “Totem”, Fragaria × ananassa Duch., ont été examinés pour déterminer l’indice de dispersion, la relation variance–moyenne, la distribution et le rapport entre le nombre moyen de T. urticae/foliole et la proportion de folioles sans T. urticae. La pente de la relation variance–moyenne, 1,64 ± 0,0355 (SE), ne diffère pas entre les dates d’échantillonnage, mais le point d’intersection diminue significativement, passant de 2,56 ± 0,0969 avant la récolte à 1,76 ± 0,140 pendant larécolte. À 0,0136 T. urticae/foliole avant et 0,0644 T. urticae/foliole pendant, la variance égale la moyenne, ce qui signifie qu’à ces densités, les données suivent la distribution de Poisson. Au-dessus de ces densités, les données sont très dispersées, la plupart des échantillons suivant la distribution bionomiale négative, mais certains tendant à montrer une plus grande dispersion que la binomiale négative. Il n’existe pas de coefficient k commun pour la binomiale négative et les données ne correspondent pas à la prévision d’un rapport 1/k conforme à la relation variance–moyenne. Un plan d’échantillonnage indépendant de la distribution, basé sur la moyenne des échantillons et la proportion de folioles sans T. urticae (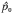), a été mis au point. On peut rapidement déterminer à l’oeil nu la densité du tétranyque en plein champ en observant itérativement les folioles pour la présence ou l’absence de T. urticae et en consultant une petite table qui donne la densité moyenne pour un 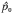donné et le nombre de folioles nécessaires pour obtenir une erreur-type particulière.

Type
Articles
Information
The Canadian Entomologist , Volume 118 , Issue 8 , August 1986 , pp. 807 - 814
Copyright
Copyright © Entomological Society of Canada 1986

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anscombe, F.J. 1950. Sampling theory of the negative binomial and logarithmic series distributions. Biometrika 37: 358–382.CrossRefGoogle ScholarPubMed
Bliss, C.I. 1967. Statistics in biology, vol. 1. McGraw-Hill, New York. 558 ppGoogle Scholar
Bliss, C.I., and Fisher, R.A.. 1953. Fitting the negative binomial distribution to biological data and note on the efficient fitting of the negative binomial. Biometrics 9: 176–200.CrossRefGoogle Scholar
Bliss, C.I., and Owen, A.R.G.. 1958. Negative binomial distributions with a common k. Biometrika 45: 37–58.CrossRefGoogle Scholar
Evans, D.A. 1953. Experimental evidence concerning contagious distributions in ecology. Biometrika 40: 186–211.CrossRefGoogle Scholar
Gerrard, D.J., and Chiang, H.C.. 1970. Density estimation of corn rootworm egg populations based upon frequency of occurrence. Ecology 51: 237–245.CrossRefGoogle Scholar
Nachman, G. 1984. Estimates of mean population density and spatial distribution of Tetranychus urticae (Acarina: Tetranychidae) and Phytoseiulus persimilis (Acarina: Phytoseiidae) based upon the proportion of empty sampling units. J. Appl. Ecol. 21: 903–913.CrossRefGoogle Scholar
Plant, R.E., and Wilson, L.T.. 1985. A Bayesian method for sequential sampling and forecasting in agricultural pest management. Biometrics 41: 203–214.CrossRefGoogle Scholar
Raworth, D.A. 1986. An economic threshold function for the twospotted spider mite, Tetranychus urticae (Acari: Tetranychidae) on strawberries. Can. Ent. 118: 9–16.CrossRefGoogle Scholar
Taylor, L.R. 1961. Aggregation, variance and the mean. Nature 189: 732–735.CrossRefGoogle Scholar
Taylor, L.R. 1965. A natural law for the spatial disposition of insects. pp. 396–397in Proceedings of the XII International Congress on Entomology.Google Scholar
Taylor, L.R., Woiwood, I.P., and Perry, J.N.. 1979. The negative binomial as a dynamic ecological model for aggregation, and the density dependence of k. J. Anim. Ecol. 48: 289–304.CrossRefGoogle Scholar
Wilson, L.T., and Room, P.M.. 1983. Clumping patterns of fruit and arthropods in cotton, with implications for binomial sampling. Environ. Ent. 12: 50–54.CrossRefGoogle Scholar