No CrossRef data available.
Published online by Cambridge University Press: 17 April 2009
Let H(C) be the group of homeomorphisms of the cantor set, C onto itself. Let p: C → M be a (continuous) map of C onto a compact metric space M, and let G(p, M) be {h ∈ H(C) | ∀x ∈ C, p(x) = ph(x)}. G(p, M) is a group. The map p: C → M is standard, if for each (x, y) ∈ C × C such that p(x) = p(y), there is a sequence 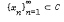 and a sequence
and a sequence  such that xn → x and hn(xn) → y. Standard maps and their associated groups characterize compact metric spaces in the sense that: Two such spaces, M and N, are homeomorphic if and only if, given p standard from C onto M, there is a standard q from C onto N for which G(p, M) = h−1G(q, N)h, for some h ∈ H(C). That is, two compact metric spaces are homeomorphic if and only if they determine, via standard maps, the same classes of conjugate subgroups of H(C).
such that xn → x and hn(xn) → y. Standard maps and their associated groups characterize compact metric spaces in the sense that: Two such spaces, M and N, are homeomorphic if and only if, given p standard from C onto M, there is a standard q from C onto N for which G(p, M) = h−1G(q, N)h, for some h ∈ H(C). That is, two compact metric spaces are homeomorphic if and only if they determine, via standard maps, the same classes of conjugate subgroups of H(C).
The present note exhibits two natural structure theorems relating algebraic and topological properties: First, if M = H ∪ K (H, K ≠ π) , compact metric, and p : C → M are given, then G(p, M) is isomorphic to a subdirect product of G(p, M)/S(p, H\K) and G(p, M)/S(p, K\H) where, generally, S(p, N) is the normal subgroup of homeomorphisms supported on p−1M . Second, given M and N compact metric and p : M → N continuous and onto, let M ≠ M − CID*α ≠ 0 , where {Dα}α ∈ A is the collection of non-degenerate preimages of points in N Then there is a standard p : C → M such that fp : C → N is standard and there is a homomorphism
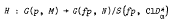 .
.