Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-19T15:38:08.554Z Has data issue: false hasContentIssue false
  • English
  • Français

STRICT TOPOLOGY AS A MIXED TOPOLOGY ON LEBESGUE SPACES

Part of: Topological linear spaces and related structures Abstract harmonic analysis

Published online by Cambridge University Press:  06 September 2011

SAEID MAGHSOUDI  and
RASOUL NASR-ISFAHANI
SAEID MAGHSOUDI*
Affiliation:
Department of Mathematics, Zanjan University, Zanjan, Iran Research Institute for Fundamental Science, Tabriz, Iran (email: [email protected])
RASOUL NASR-ISFAHANI
Affiliation:
Department of Mathematical Sciences, Isfahan University of Technology, Isfahan 84156-83111, Iran (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let X be a locally compact space, and 𝔏∞0(X,ι) be the space of all essentially bounded ι-measurable functions f on X vanishing at infinity. We introduce and study a locally convex topology β1(X,ι) on the Lebesgue space 𝔏1(X,ι) such that the strong dual of (𝔏1(X,ι),β1(X,ι)) can be identified with 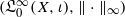. Next, by showing that β1(X,ι) can be considered as a natural mixed topology, we deduce some of its basic properties. Finally, as an application, we prove that L1 (G) , the group algebra of a locally compact Hausdorff topological group G, equipped with the convolution multiplication is a complete semitopological algebra under the β1 (G) topology.

Keywords

group algebrasLebesgue spaceslocally compact grouplocally convex topologymixed topologyRadon measurestrict topology

MSC classification

Secondary: 46A03: General theory of locally convex spaces 46A70: Saks spaces and their duals (strict topologies, mixed topologies, two-norm spaces, co-Saks spaces, etc.) 43A20: $L^1$-algebras on groups, semigroups, etc.
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 84 , Issue 3 , December 2011 , pp. 504 - 515
Copyright
Copyright © Australian Mathematical Publishing Association Inc. 2011

References

[1]Conradie, J., ‘Mackey topologies and mixed topologies in Riesz spaces’, Positivity 10 (2006), 591–606.CrossRefGoogle Scholar
[2]Cooper, J. B., Saks Spaces and Applications to Functional Analysis, 2nd edn North-Holland Mathematics Studies, 139 (North-Holland, Amsterdam, 1987).Google Scholar
[3]Dales, H. G., Banach Algebras and Automatic Continuity, London Mathematical Society Monographs, 24 (Oxford University Press, New York, 2000).Google Scholar
[4]Grothendieck, A., Produits tensoriels topologiques et espaces uncléaires, Memoirs of the American Mathematical Society, 16 (American Mathematical Society, Providence, RI, 1955).Google Scholar
[5]Gulick, D., ‘Duality theory for the strict topology’, Studia Math. 49 (1974), 195–208.CrossRefGoogle Scholar
[6]Hewitt, E. and Ross, K., Abstract Harmonic Analysis I (Springer, New York, 1970).Google Scholar
[7]Hewitt, E. and Stromberg, K., Real and Abstract Analysis (Springer, New York, 1975).Google Scholar
[8]Kasior, E. and Nowak, M., ‘Generalized mixed topology on F-normed function spaces’, Comment. Math. (Prace Mat.) 44 (2004), 55–66.Google Scholar
[9]Maghsoudi, S. and Nasr-Isfahani, R., ‘Strict topology on the discrete Lebesgue spaces’, Bull. Aust. Math. Soc. 83 (2011), 241–255.CrossRefGoogle Scholar
[10]Maghsoudi, S., Nasr-Isfahani, R. and Rejali, A., ‘Strong Arens irregularity of Beurling algebras with a locally convex topology’, Arch. Math. (Basel) 86 (2006), 437–448.CrossRefGoogle Scholar
[11]Maghsoudi, S., Nasr-Isfahani, R. and Rejali, A., ‘The dual of semigroup algebras with certain locally convex topologies’, Semigroup Forum 73 (2006), 367–376.CrossRefGoogle Scholar
[12]Robertson, J. M. and Wiser, H. C., ‘A note on polar topologies’, Canad. Math. Bull. 11 (1968), 607–609.CrossRefGoogle Scholar
[13]Singh, A. I., ‘L ∞0(G)* as the second dual of the group algebra L 1(G) with a locally convex topology’, Michigan Math. J. 46 (1999), 143–150.CrossRefGoogle Scholar
[14]Swartz, C., An Introduction to Functional Analysis, Pure and Applied Mathematics, 157 (Marcel Dekker, New York, 1992).Google Scholar
[15]Wiweger, A., ‘Linear spaces with mixed topology’, Studia Math. 20 (1961), 47–68.CrossRefGoogle Scholar