No CrossRef data available.
Published online by Cambridge University Press: 27 December 2023
Suppose that G is a finite solvable group. Let 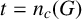 $t=n_c(G)$ denote the number of orders of nonnormal subgroups of G. We bound the derived length
$t=n_c(G)$ denote the number of orders of nonnormal subgroups of G. We bound the derived length 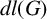 $dl(G)$ in terms of
$dl(G)$ in terms of  $n_c(G)$. If G is a finite p-group, we show that
$n_c(G)$. If G is a finite p-group, we show that 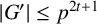 $|G'|\leq p^{2t+1}$ and
$|G'|\leq p^{2t+1}$ and 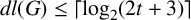 $dl(G)\leq \lceil \log _2(2t+3)\rceil $. If G is a finite solvable nonnilpotent group, we prove that the sum of the powers of the prime divisors of
$dl(G)\leq \lceil \log _2(2t+3)\rceil $. If G is a finite solvable nonnilpotent group, we prove that the sum of the powers of the prime divisors of 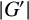 $|G'|$ is less than t and that
$|G'|$ is less than t and that  $dl(G)\leq \lfloor 2(t+1)/3\rfloor +1$.
$dl(G)\leq \lfloor 2(t+1)/3\rfloor +1$.
This research is supported by the National Natural Science Foundation of China (Nos. 11971391, 12071376), by Fundamental Research Funds for the Central Universities (SWU-XDJH202305) and the Postgraduate Research and Innovation Project of Southwest University (SWUB23034).