Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-01-10T23:38:41.315Z Has data issue: false hasContentIssue false
  • English
  • Français

THE SECOND SHIFTED DIFFERENCE OF PARTITIONS AND ITS APPLICATIONS

Part of: Additive number theory; partitions

Published online by Cambridge University Press:  25 August 2022

KEVIN GOMEZ Open the ORCID record for KEVIN GOMEZ [Opens in a new window] ,
JOSHUA MALES Open the ORCID record for JOSHUA MALES [Opens in a new window]  and
LARRY ROLEN Open the ORCID record for LARRY ROLEN [Opens in a new window]
KEVIN GOMEZ
Affiliation:
Department of Mathematics, Vanderbilt University, 1420 Stevenson Center, Nashville, TN 37240, USA e-mail: [email protected]
JOSHUA MALES*
Affiliation:
Department of Mathematics, University of Manitoba, 450 Machray Hall, Winnipeg, Canada
LARRY ROLEN
Affiliation:
Department of Mathematics, Vanderbilt University, 1420 Stevenson Center, Nashville, TN 37240, USA e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A number of recent papers have estimated ratios of the partition function 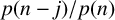$p(n-j)/p(n)$ , which appear in many applications. Here, we prove an easy-to-use effective bound on these ratios. Using this, we then study the second shifted difference of partitions, 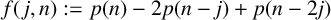$f(\,j,n) := p(n) -2p(n-j) +p(n-2j)$ , and give another easy-to-use estimate of 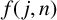$f(\,j,n)$ . As applications of these, we prove a shifted convexity property of 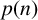$p(n)$ , as well as giving new estimates of the k-rank partition function 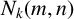$N_k(m,n)$ and non-k-ary partitions along with their differences.

Keywords

partitionconvexityasymptotic expansion

MSC classification

Primary: 11P82: Analytic theory of partitions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 107 , Issue 1 , February 2023 , pp. 66 - 78
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The research of the second author conducted for this paper is supported by the Pacific Institute for the Mathematical Sciences (PIMS). The research and findings may not reflect those of the Institute. This work was supported by a grant from the Simons Foundation (853830, LR). The third author is also grateful for support from a 2021–2023 Dean’s Faculty Fellowship from Vanderbilt University and to the Max Planck Institute for Mathematics in Bonn for its hospitality and financial support.

References

Akande, A., Genao, T., Haag, S., Hendon, M., Pulagam, N., Schneider, R. and Sills, A., ‘Computational study of non-unitary partitions’, Preprint, 2021, arXiv:2112.03264.Google Scholar
Bringmann, K., Kane, B., Rolen, L. and Tripp, Z., ‘Fractional partitions and conjectures of Chern–Fu–Tang and Heim–Neuhauser’, Trans. Amer. Math. Soc. Ser. B 8 (2021), 615–634.CrossRefGoogle Scholar
Canfield, R., Corteel, S. and Hitczenko, P., ‘Random partitions with non-negative $r$ -th differences’, Adv. Appl. Math. 27(2–3) (2001), 298–317. Special issue in honor of Dominique Foata’s 65th birthday (Philadelphia, PA, 2000).CrossRefGoogle Scholar
Chen, W. Y. C., Wang, L. X. W. and Xie, G. Y. B., ‘Finite differences of the logarithm of the partition function’, Math. Comp. 85(298) (2016), 825–847.CrossRefGoogle Scholar
Garvan, F., ‘Generalizations of Dyson’s rank and non-Rogers–Ramanujan partitions’, Manuscripta Math. 84(3–4) (1994), 343–359.CrossRefGoogle Scholar
Griffin, M., Ono, K., Rolen, L. and Zagier, D., ‘Jensen polynomials for the Riemann zeta function and other sequences’, Proc. Natl. Acad. Sci. USA 116(23) (2019), 11103–11110.CrossRefGoogle ScholarPubMed
Gupta, H., ‘Finite differences of the partition function’, Math. Comp. 32(144) (1978), 1241–1243.CrossRefGoogle Scholar
Hardy, G. and Ramanujan, S., ‘Asymptotic formulae in combinatory analysis’, Proc. Lond. Math. Soc. (2) 17 (1918), 75–115.CrossRefGoogle Scholar
Iskander, J., Jain, V. and Talvola, V., ‘Exact formulae for the fractional partition functions’, Res. Number Theory 6 (2020), 201–215.CrossRefGoogle Scholar
Knessl, C., ‘Asymptotic behavior of high-order differences of the plane partition function’, Discrete Math. 126(1–3) (1994), 179–193.CrossRefGoogle Scholar
Knessl, C. and Keller, J. B., ‘Asymptotic behavior of high-order differences of the partition function’, Comm. Pure Appl. Math. 44(8–9) (1991), 1033–1045.CrossRefGoogle Scholar
Larson, H. and Wagner, I., ‘Hyperbolicity of the partition Jensen polynomials’, Res. Number Theory 5(2) (2019), Article no. 19, 12 pages.Google Scholar
Lehmer, D., ‘On the series for the partition function’, Trans. Amer. Math. Soc. 43(2) (1938), 271–295.CrossRefGoogle Scholar
Lehmer, D., ‘On the remainders and convergence of the series for the partition function’, Trans. Amer. Math. Soc. 46 (1939), 362–373.CrossRefGoogle Scholar
Liu, Z. and Zhou, N. H., ‘Uniform asymptotic formulas for the Fourier coefficients of the inverse of theta functions’, Ramanujan J. 57 (2022), 1085–1123.CrossRefGoogle Scholar
Merca, M. and Katriel, J., ‘A general method for proving the non-trivial linear homogeneous partition inequalities’, Ramanujan J. 51(2) (2020), 245–266.CrossRefGoogle Scholar
Odlyzko, A. M., ‘Differences of the partition function’, Acta Arith. 49(3) (1988), 237–254.CrossRefGoogle Scholar
Rademacher, H., ‘ A convergent series for the partition function $p(n)$ ’, Proc. Natl. Acad. Sci. USA 23(2) (1937), 78–84.CrossRefGoogle ScholarPubMed
Schneider, R., ‘Nuclear partitions and a formula for $p(n)$ ’, Preprint, 2020, arXiv:1912.00575.Google Scholar
Watson, G., A Treatise on the Theory of Bessel Functions (Cambridge University Press, Cambridge, 1995).Google Scholar