Hostname: page-component-745bb68f8f-hvd4g Total loading time: 0 Render date: 2025-02-05T03:46:13.478Z Has data issue: false hasContentIssue false
  • English
  • Français

PROOF OF A CONJECTURE OF BANERJEE AND DASTIDAR ON ODD CRANK

Part of: Additive number theory; partitions Combinatorics

Published online by Cambridge University Press:  13 January 2023

DAZHAO TANG Open the ORCID record for DAZHAO TANG [Opens in a new window]
DAZHAO TANG*
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 401331, PR China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Recently, when studying intricate connections between Ramanujan’s theta functions and a class of partition functions, Banerjee and Dastidar [‘Ramanujan’s theta functions and parity of parts and cranks of partitions’, Ann. Comb., to appear] studied some arithmetic properties for 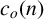$c_o(n)$, the number of partitions of n with odd crank. They conjectured a congruence modulo 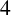$4$ satisfied by 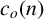$c_o(n)$. We confirm the conjecture and evaluate 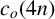$c_o(4n)$ modulo $8$ by dissecting some q-series into even powers. Moreover, we give a conjecture on the density of divisibility of odd cranks modulo 4, 8 and 16.

Keywords

partitionscongruencesodd crankJacobi’s identityJacobi’s triple product identity

MSC classification

Primary: 11P83: Partitions; congruences and congruential restrictions
Secondary: 05A17: Partitions of integers
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 108 , Issue 3 , December 2023 , pp. 406 - 411
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work was partially supported by the National Natural Science Foundation of China (No. 12201093), the Natural Science Foundation Project of Chongqing CSTB (No. CSTB2022NSCQ–MSX0387), the Science and Technology Research Program of Chongqing Municipal Education Commission (No. KJQN202200509) and the Doctoral start-up research grant (No. 21XLB038) of Chongqing Normal University.

References

Andrews, G. E. and Berndt, B. C., Ramanujan’s Lost Notebook. Part I (Springer, New York, 2005).10.1007/0-387-28124-XCrossRefGoogle Scholar
Andrews, G. E., Berndt, B. C., Chan, S. H., Kim, S. and Malik, A., ‘Four identities and third order mock theta functions’, Nagoya Math. J. 239 (2020), 1–32.10.1017/nmj.2018.35CrossRefGoogle Scholar
Andrews, G. E. and Garvan, F. G., ‘Dyson’s crank of a partition’, Bull. Amer. Math. Soc. (N.S.) 18(2) (1988), 167–171.10.1090/S0273-0979-1988-15637-6CrossRefGoogle Scholar
Atkin, A. O. L. and Swinnerton-Dyer, P., ‘Some properties of partitions’, Proc. Lond. Math. Soc. (3) 4 (1954), 84–106.10.1112/plms/s3-4.1.84CrossRefGoogle Scholar
Banerjee, K. and Dastidar, M. G., ‘Ramanujan’s theta functions and parity of parts and cranks of partitions’, Ann. Comb., to appear. Published online (25 October 2022).10.1007/s00026-022-00615-1CrossRefGoogle Scholar
Berndt, B. C., Number Theory in the Spirit of Ramanujan, Student Mathematical Library, 34 (American Mathematical Society, Providence, RI, 2006).10.1090/stml/034CrossRefGoogle Scholar
Dyson, F. J., ‘Some guesses in the theory of partitions’, Eureka (Cambridge) 8 (1944), 10–15.Google Scholar
Ramanujan, S., ‘Congruence properties of partitions’, Proc. Lond. Math. Soc. (3) 19(2) (1919), 207–210.Google Scholar