Hostname: page-component-f554764f5-c4bhq Total loading time: 0 Render date: 2025-04-11T01:47:36.286Z Has data issue: false hasContentIssue false
  • English
  • Français

PERIODS OF DUCCI SEQUENCES AND ODD SOLUTIONS TO A PELLIAN EQUATION

Part of: Sequences and sets Algebraic number theory: global fields Diophantine equations

Published online by Cambridge University Press:  03 July 2019

FLORIAN BREUER Open the ORCID record for FLORIAN BREUER [Opens in a new window]
FLORIAN BREUER*
Affiliation:
University of Newcastle, Callaghan, NSW 2308, Australia email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A Ducci sequence is a sequence of integer $n$-tuples generated by iterating the map

$$\begin{eqnarray}D:(a_{1},a_{2},\ldots ,a_{n})\mapsto (|a_{1}-a_{2}|,|a_{2}-a_{3}|,\ldots ,|a_{n}-a_{1}|).\end{eqnarray}$$
Such a sequence is eventually periodic and we denote by $P(n)$ the maximal period of such sequences for given $n$. We prove a new upper bound in the case where $n$ is a power of a prime 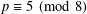$p\equiv 5\hspace{0.6em}({\rm mod}\hspace{0.2em}8)$ for which 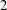$2$ is a primitive root and the Pellian equation $x^{2}-py^{2}=-4$ has no solutions in odd integers $x$ and $y$.

Keywords

Ducci sequencePellian equationcyclotomic fieldreal quadratic unit

MSC classification

Primary: 11B83: Special sequences and polynomials
Secondary: 11D09: Quadratic and bilinear equations 11R18: Cyclotomic extensions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 100 , Issue 2 , October 2019 , pp. 201 - 205
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© 2019 Australian Mathematical Publishing Association Inc.

References

Arnold, V. I., ‘Complexity of finite sequences of zeroes and ones and geometry of finite spaces of functions’, Funct. Anal. Other Math. 1 (2006), 1–15.10.1007/s11853-007-0001-0Google Scholar
Behn, A., Kribs-Zaleta, C. and Ponomarenko, V., ‘The convergence of difference boxes’, Amer. Math. Monthly 112(5) (2005), 426–439.10.1080/00029890.2005.11920211Google Scholar
Berlekamp, E. R., ‘The design of slowly shrinking labelled squares’, Math. Comp. 29 (1975), 25–27.10.1090/S0025-5718-1975-0373933-6Google Scholar
Breuer, F., ‘Ducci sequences and cyclotomic fields’, J. Difference Equ. Appl. 16(7) (2010), 847–862.10.1080/10236190802566509Google Scholar
Breuer, F., Lötter, E. and van der Merwe, A. B., ‘Ducci sequences and cyclotomic polynomials’, Finite Fields Appl. 13 (2007), 293–304.Google Scholar
Brockman, G. and Zerr, R. J., ‘Asymptotic behaviour of certain Ducci sequences’, Fibonacci Quart. 45(2) (2007), 155–163.Google Scholar
Burmester, M., Forcade, R. and Jacobs, E., ‘Circles of numbers’, Glasg. Math. J. 19 (1978), 115–119.10.1017/S0017089500003487Google Scholar
Ciamberlini, C. and Marengoni, A., ‘Su una interessante curiosità numerica’, Periodiche di Matematiche 17 (1937), 25–30.Google Scholar
Clausing, A., ‘Ducci matrices’, Amer. Math. Monthly 125(10) (2018), 901–921.Google Scholar
Cohen, H., Number Theory, Volume I: Tools and Diophantine Equations, Graduate Texts in Mathematics, 239 (Springer, New York, 2007).Google Scholar
Ehrlich, A., ‘Periods of Ducci’s N-number game of differences’, Fibonacci Quart. 28(4) (1990), 302–305.Google Scholar
Freedman, B., ‘The four number game’, Scripta Math. 14 (1948), 35–47.Google Scholar
Hartung, P. G., ‘On the Pellian equation’, J. Number Theory 12(1) (1980), 110–112.10.1016/0022-314X(80)90080-3Google Scholar
Moree, P., ‘On primes in arithmetic progression having a prescribed primitive root. II’, Funct. Approx. Comment. Math. 39 (2008), 133–144.10.7169/facm/1229696559Google Scholar
On-line Encyclopedia of Integer Sequences, entry $\#$ A130229, https://oeis.org/A130229.Google Scholar
Simmons, G. J., ‘The structure of the differentiation digraphs of binary sequences’, Ars Combin. 35A (1993), 71–88.Google Scholar
Stevenhagen, P., ‘On a problem of Eisenstein’, Acta Arith. 74(3) (1996), 259–268.10.4064/aa-74-3-259-268Google Scholar
Zvengrowski, P., ‘Iterated absolute differences’, Math. Mag. 52(1) (1979), 36–40.10.1080/0025570X.1979.11976749Google Scholar