Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mann, Avinoam
1999.
Some questions aboutp-groups.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 67,
Issue. 3,
p.
356.
Mann, Avinoam
2000.
The Derived Length of p-Groups.
Journal of Algebra,
Vol. 224,
Issue. 2,
p.
263.
Evans-Riley, Susan
2001.
On the derived length of finite, graded Lie rings with prime-power order, and groups with prime-power order.
Bulletin of the Australian Mathematical Society,
Vol. 64,
Issue. 1,
p.
171.
Schneider, Csaba
2001.
Some results on the derived series of finite p-groups.
Bulletin of the Australian Mathematical Society,
Vol. 63,
Issue. 2,
p.
351.
Schneider, Csaba
2003.
Groups of prime-power order with a small second derived quotient.
Journal of Algebra,
Vol. 266,
Issue. 2,
p.
539.
Schneider, Csaba
2007.
The derived series of a finite p-group.
Journal of Algebra,
Vol. 307,
Issue. 1,
p.
136.
Conder, Marston
Exoo, Geoffrey
and
Jajcay, Robert
2010.
On the limitations of the use of solvable groups in Cayley graph cage constructions.
European Journal of Combinatorics,
Vol. 31,
Issue. 7,
p.
1819.
CRESTANI, ELEONORA
and
LUCCHINI, ANDREA
2016.
LARGE P-GROUPS WITHOUT PROPER SUBGROUPS WITH THE SAME DERIVED LENGTH.
Glasgow Mathematical Journal,
Vol. 58,
Issue. 2,
p.
445.


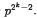 .
.