Article contents
ON THE DIVISIBILITY AMONG POWER LCM MATRICES ON GCD-CLOSED SETS
Published online by Cambridge University Press: 19 May 2022
Abstract
Let
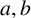 $a,b$
and n be positive integers and let
$a,b$
and n be positive integers and let
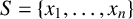 $S=\{x_1, \ldots , x_n\}$
be a set of n distinct positive integers. For
$S=\{x_1, \ldots , x_n\}$
be a set of n distinct positive integers. For
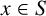 ${x\in S}$
, define
${x\in S}$
, define
 $G_{S}(x)=\{d\in S: d<x, \,d\mid x \ \mathrm {and} \ (d\mid y\mid x, y\in S)\Rightarrow y\in \{d,x\}\}$
. Denote by
$G_{S}(x)=\{d\in S: d<x, \,d\mid x \ \mathrm {and} \ (d\mid y\mid x, y\in S)\Rightarrow y\in \{d,x\}\}$
. Denote by
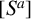 $[S^a]$
the
$[S^a]$
the
 $n\times n$
matrix having the ath power of the least common multiple of
$n\times n$
matrix having the ath power of the least common multiple of
 $x_i$
and
$x_i$
and
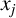 $x_j$
as its
$x_j$
as its
 $(i,j)$
-entry. We show that the bth power matrix
$(i,j)$
-entry. We show that the bth power matrix
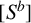 $[S^b]$
is divisible by the ath power matrix
$[S^b]$
is divisible by the ath power matrix
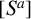 $[S^a]$
if
$[S^a]$
if
 $a\mid b$
and S is gcd closed (that is,
$a\mid b$
and S is gcd closed (that is,
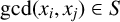 $\gcd (x_i, x_j)\in S$
for all integers i and j with
$\gcd (x_i, x_j)\in S$
for all integers i and j with
 $1\le i, j\le n$
) and
$1\le i, j\le n$
) and
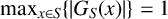 $\max _{x\in S} \{|G_S (x)|\}=1$
. This confirms a conjecture of Shaofang Hong [‘Divisibility properties of power GCD matrices and power LCM matrices’, Linear Algebra Appl. 428 (2008), 1001–1008].
$\max _{x\in S} \{|G_S (x)|\}=1$
. This confirms a conjecture of Shaofang Hong [‘Divisibility properties of power GCD matrices and power LCM matrices’, Linear Algebra Appl. 428 (2008), 1001–1008].
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 107 , Issue 1 , February 2023 , pp. 31 - 39
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
References
- 3
- Cited by



