No CrossRef data available.
Published online by Cambridge University Press: 17 April 2009
In this paper, we study a nonlinear partial differential equation on a compact manifold;
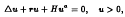
where a > 1 is a constant, r is a positive constant, and H is a prescribed smooth function.
Kazdan and Warner showed that if λ1(g) < 0 and 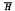 < 0, where
< 0, where 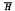 is the mean of H, then there is a constant 0 < r0(H) ≤ ∞ such that one can solve this equation for 0 < r < r0(H), but not for r > r0(H). They also proved that if r0(H) = ∞, then H(x) ≤ 0 (≢0) for all x ∈ M. They conjectured that this necessary condition might be sufficient.
is the mean of H, then there is a constant 0 < r0(H) ≤ ∞ such that one can solve this equation for 0 < r < r0(H), but not for r > r0(H). They also proved that if r0(H) = ∞, then H(x) ≤ 0 (≢0) for all x ∈ M. They conjectured that this necessary condition might be sufficient.
I show that this conjecture is right; that is, if H(x) ≤ 0 (≠ 0) for all x ∈ M, then r0(H) = ∞.