Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2025-01-03T17:32:39.213Z Has data issue: false hasContentIssue false
  • English
  • Français

On starlike functions

Published online by Cambridge University Press:  17 April 2009

V.P. Gupta  and
Iqbal Ahmad
V.P. Gupta
Affiliation:
Department of Mathematics, National Council of Educational Research and Training, New Delhi 110016, India
Iqbal Ahmad
Affiliation:
Department of Mathematics, Faculty of Humanities and Sciences, Jamia Millia Islamia, New Delhi 110025, India.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let S denote the class of functions f analytic and univalent in the open disc {z: |z| < 1} and normalized by f(0) = 0 = f′(0) − 1, and S*(α) denote the set of starlike functions of order α (0 ≤ α ≤ 1) in S. In this paper, the results of William M. Causey and William L. White [J. Math. Anal. Appl. 64 (1978), 458–466] are generalized by the following:

THEOREM 1. Let f, g and h belong to S*(α), S*(λ) and S*(γ) , respectively and let F be defined by

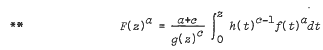
where a, c ∈ N. Then F belongs to S*(β) for |z| less than a suitably chosen number.

THEOREM 2. Let F, g and h belong to S*(α), S*(λ) and S*(γ) , respectively and f be given by **. Then f belongs to S*(β) , for |z| less than a suitably chosen number.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 22 , Issue 2 , October 1980 , pp. 241 - 247
Copyright
Copyright © Australian Mathematical Society 1980

References

[1]Bernardi, S.D., “Convex and starlike univalent functions”, Trans. Amer. Math. Soc. 135 (1969), 429–446.CrossRefGoogle Scholar
[2]Bernardi, S.D., “The radius of univalence of certain analytic functions”, Proc. Amer. Math. Soc. 24 (1970), 312–318.CrossRefGoogle Scholar
[3]Causey, William M. and White, William L., “Starlikeness of certain functions with integral representations”, J. Math. Anal. Appl. 64 (1978), 458–466.CrossRefGoogle Scholar
[4]Gupta, Ved. P., Jain, Pawan K., “On starlike functions”, Rend. Mat. (6) 9 (1976), 433–437.Google Scholar
[5]Karunakaran, V., “Certain classes of regular univalent functions”, Pacific J. Math. 61 (1975), 173–182.CrossRefGoogle Scholar
[6]Libera, R.J., “Some classes of regular univalent functions”, Proc. Amer. Math. Soc. 19 (1965), 755–758.CrossRefGoogle Scholar
[7]Livingston, A.E., “On the radius of univalence of certain analytic functions”, Proc. Amer. Math. Soc. 17 (1966), 352–357.CrossRefGoogle Scholar
[8]Padmanabhan, K.S., “On the radius of univalence of certain classes of analytic functions”, J. London Math. Soc. (2) 1 (1969), 225–231.CrossRefGoogle Scholar
[9]Singh, Ram, “On Bazilevic functions”, Proc. Amer. Math. Soc. 38 (1973), 261–271.Google Scholar