Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-22T22:12:44.851Z Has data issue: false hasContentIssue false
  • English
  • Français

ON NUMBERS $n$ WITH POLYNOMIAL IMAGE COPRIME WITH THE $n$TH TERM OF A LINEAR RECURRENCE

Part of: Sequences and sets Multiplicative number theory Elementary number theory

Published online by Cambridge University Press:  28 August 2018

DANIELE MASTROSTEFANO Open the ORCID record for DANIELE MASTROSTEFANO [Opens in a new window]  and
CARLO SANNA Open the ORCID record for CARLO SANNA [Opens in a new window]
DANIELE MASTROSTEFANO
Affiliation:
Mathematics Institute, Zeeman Building, University of Warwick, Coventry, CV4 7AL, UK email [email protected]
CARLO SANNA*
Affiliation:
Department of Mathematics, Università degli Studi di Torino, Turin, Italy email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $F$ be an integral linear recurrence, $G$ an integer-valued polynomial splitting over the rationals and 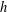$h$ a positive integer. Also, let 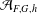${\mathcal{A}}_{F,G,h}$ be the set of all natural numbers $n$ such that 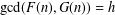$\gcd (F(n),G(n))=h$. We prove that 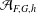${\mathcal{A}}_{F,G,h}$ has a natural density. Moreover, assuming that 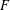$F$ is nondegenerate and $G$ has no fixed divisors, we show that the density of ${\mathcal{A}}_{F,G,1}$ is 0 if and only if 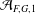${\mathcal{A}}_{F,G,1}$ is finite.

Keywords

greatest common divisorlinear recurrencesnatural densities

MSC classification

Primary: 11B37: Recurrences
Secondary: 11A07: Congruences; primitive roots; residue systems 11B39: Fibonacci and Lucas numbers and polynomials and generalizations 11N25: Distribution of integers with specified multiplicative constraints
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 99 , Issue 1 , February 2019 , pp. 23 - 33
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

The second author is a member of INdAM group GNSAGA.

References

Ailon, N. and Rudnick, Z., ‘Torsion points on curves and common divisors of a k - 1 and b k - 1’, Acta Arith. 113(1) (2004), 31–38.Google Scholar
Alba González, J. J., Luca, F., Pomerance, C. and Shparlinski, I. E., ‘On numbers n dividing the nth term of a linear recurrence’, Proc. Edinb. Math. Soc. (2) 55(2) (2012), 271–289.Google Scholar
André-Jeannin, R., ‘Divisibility of generalized Fibonacci and Lucas numbers by their subscripts’, Fibonacci Quart. 29(4) (1991), 364–366.Google Scholar
Bugeaud, Y., Corvaja, P. and Zannier, U., ‘An upper bound for the G.C.D. of a n - 1 and b n - 1’, Math. Z. 243(1) (2003), 79–84.Google Scholar
Corvaja, P. and Zannier, U., ‘Diophantine equations with power sums and universal Hilbert sets’, Indag. Math. (N.S.) 9(3) (1998), 317–332.Google Scholar
Corvaja, P. and Zannier, U., ‘Finiteness of integral values for the ratio of two linear recurrences’, Invent. Math. 149(2) (2002), 431–451.Google Scholar
Everest, G. and Harman, G., ‘On primitive divisors of n 2 + b ’, in: Number Theory and Polynomials, London Mathematical Society Lecture Note Series, 352 (eds. McKee, J. and Smyth, C.) (Cambridge University Press, Cambridge, 2008), 142–154.Google Scholar
Everest, G., van der Poorten, A., Shparlinski, I. and Ward, T., Recurrence Sequences, Mathematical Surveys and Monographs, 104 (American Mathematical Society, Providence, RI, 2003).Google Scholar
Fuchs, C., ‘An upper bound for the G.C.D. of two linear recurring sequences’, Math. Slovaca 53(1) (2003), 21–42.Google Scholar
Greaves, G., Sieves in Number Theory, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), 43 (Springer, Berlin, 2001).Google Scholar
Kim, S., ‘The density of the terms in an elliptic divisibility sequence having a fixed G.C.D. with their index’, Preprint, 2017, arXiv:1708.08357.Google Scholar
Leonetti, P. and Sanna, C., ‘On the greatest common divisor of n and the nth Fibonacci number’, Rocky Mountain J. Math. to appear.Google Scholar
Luca, F. and Tron, E., ‘The distribution of self-Fibonacci divisors’, in: Advances in the Theory of Numbers, Fields Institute Communications, 77 (Fields Institute for Research in Mathematical Sciences, Toronto, ON, 2015), 149–158.Google Scholar
Sanna, C., ‘Distribution of integral values for the ratio of two linear recurrences’, J. Number Theory 180 (2017), 195–207.Google Scholar
Sanna, C., ‘On numbers n dividing the nth term of a Lucas sequence’, Int. J. Number Theory 13(3) (2017), 725–734.Google Scholar
Sanna, C., ‘The moments of the logarithm of a G.C.D. related to Lucas sequences’, J. Number Theory 191 (2018), 305–315.Google Scholar
Sanna, C., ‘On numbers n relatively prime to the nth term of a linear recurrence’, Bull. Malays. Math. Sci. Soc. (online ready), https://doi.org/10.1007/s40840-017-0514-8.Google Scholar
Sanna, C. and Tron, E., ‘The density of numbers n having a prescribed G.C.D. with the nth Fibonacci number’, Indag. Math. (N.S.) 29(3) (2018), 972–980.Google Scholar
Schlickewei, H. P., ‘Multiplicities of recurrence sequences’, Acta Math. 176(2) (1996), 171–243.Google Scholar
Shparlinski, I. E., ‘The number of different prime divisors of recurrent sequences’, Mat. Zametki 42(4) (1987), 494–507, 622; English translation, Math. Notes 42(4) (1987), 773–780.Google Scholar
Smyth, C., ‘The terms in Lucas sequences divisible by their indices’, J. Integer Seq. 13(2) (2010), Article 10.2.4, 18 pages.Google Scholar
Somer, L., ‘Divisibility of terms in Lucas sequences by their subscripts’, in: Applications of Fibonacci Numbers, Vol. 5 (St. Andrews, 1992) (Kluwer Academic, Dordrecht, 1993), 515–525.Google Scholar
van der Poorten, A. J., ‘Solution de la conjecture de Pisot sur le quotient de Hadamard de deux fractions rationnelles’, C. R. Acad. Sci. Paris Sér. I Math. 306(3) (1988), 97–102.Google Scholar