Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-05T12:50:34.809Z Has data issue: false hasContentIssue false
  • English
  • Français

ON NONCOMMUTING SETS AND CENTRALISERS IN INFINITE GROUPS

Part of: Special aspects of infinite or finite groups Representation theory of groups

Published online by Cambridge University Press:  08 July 2015

MOHAMMAD ZARRIN
MOHAMMAD ZARRIN*
Affiliation:
Department of Mathematics, University of Kurdistan, PO Box 416, Sanandaj, Iran email [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A subset $X$ of a group $G$ is a set of pairwise noncommuting elements if 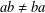$ab\neq ba$ for any two distinct elements $a$ and 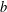$b$ in 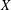$X$. If 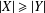$|X|\geq |Y|$ for any other set of pairwise noncommuting elements 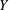$Y$ in 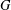$G$, then 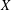$X$ is called a maximal subset of pairwise noncommuting elements and the cardinality of such a subset (if it exists) is denoted by 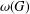${\it\omega}(G)$. In this paper, among other things, we prove that, for each positive integer $n$, there are only finitely many groups 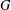$G$, up to isoclinism, with 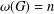${\it\omega}(G)=n$, and we obtain similar results for groups with exactly $n$ centralisers.

Keywords

pairwise noncommuting elements of a groupn-centralisers

MSC classification

Primary: 20D60: Arithmetic and combinatorial problems
Secondary: 20F99: None of the above, but in this section
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 93 , Issue 1 , February 2016 , pp. 42 - 46
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Abdollahi, A., Amiri, S. M. J. and Hassanabadi, A. M., ‘Groups with specific number of centralizers’, Houston J. Math. 33 (2007), 43–57.Google Scholar
Abdollahi, A., Azad, A., Hassanabadi, A. M. and Zarrin, M., ‘On the clique numbers of non-commuting graphs of certain groups’, Algebra Colloq. 17 (2010), 611–620.CrossRefGoogle Scholar
Amiri, S. M. J., Madadi, H. and Rostami, H., ‘On 9-centralizer groups’, J. Algebra Appl. 14(1) (2015), 1550003 (13 pages).Google Scholar
Ashrafi, A. R., ‘On finite groups with a given number of centralizers’, Algebra Colloq. 7 (2000), 139–146.CrossRefGoogle Scholar
Ashrafi, A. R., ‘Counting the centralizers of some finite groups’, Korean J. Comput. Appl. Math. 7(1) (2000), 115–124.CrossRefGoogle Scholar
Ballester-Bolinches, A. and Cossey, J., ‘On non-commuting sets in finite soluble CC-groups’, Publ. Mat. 56(2) (2012), 467–471.CrossRefGoogle Scholar
Belcastro, S. M. and Sherman, G. J., ‘Counting centralizers in finite groups’, Math. Mag. 5 (1994), 111–114.Google Scholar
Chin, A. Y. M., ‘On non-commuting sets in an extraspecial p-group’, J. Group Theory 8 (2005), 189–194.CrossRefGoogle Scholar
Endimioni, G., ‘Groupes finis satisfaisant la condition (N; n)’, C. R. Acad. Sci. Paris Ser. I 319 (1994), 1245–1247; (in French).Google Scholar
Hall, P., ‘The classification of prime power groups’, J. reine angew. Math. 182 (1940), 130–141.CrossRefGoogle Scholar
Neumann, B. H., ‘A problem of Paul Erdős on groups’, J. Aust. Math. Soc. Ser. A 21 (1976), 467–472.CrossRefGoogle Scholar
Pyber, L., ‘The number of pairwise non-commuting elements and the index of the centre in a finite group’, J. Lond. Math. Soc. (2) 35(2) (1987), 287–295.CrossRefGoogle Scholar
Zarrin, M., ‘On element-centralizers in finite groups’, Arch. Math. (Basel) 93 (2009), 497–503.CrossRefGoogle Scholar
Zarrin, M., ‘Criteria for the solubility of finite groups by its centralizers’, Arch. Math. (Basel) 96 (2011), 225–226.CrossRefGoogle Scholar
Zarrin, M., ‘On solubility of groups with finitely many centralizers’, Bull. Iranian Math. Soc. 39 (2013), 517–521.Google Scholar
Zarrin, M., ‘Derived length and centralizers of groups’, J. Algebra Appl. 14(8) (2015), 1550133 (4 pages).CrossRefGoogle Scholar