Hostname: page-component-669899f699-tpknm Total loading time: 0 Render date: 2025-04-25T12:31:39.111Z Has data issue: false hasContentIssue false
  • English
  • Français

ON CONSECUTIVE RESIDUES AND NONRESIDUES UNDER A LINEAR MAP IN A FINITE FIELD

Part of: Elementary number theory Finite fields and commutative rings (number-theoretic aspects)

Published online by Cambridge University Press:  11 October 2024

EMILY BERGMAN Open the ORCID record for EMILY BERGMAN [Opens in a new window] ,
ROBERT S. COULTER Open the ORCID record for ROBERT S. COULTER [Opens in a new window]  and
BRADLEY FAIN Open the ORCID record for BRADLEY FAIN [Opens in a new window]
EMILY BERGMAN
Affiliation:
600 Seena Road, Essex, MD 21221, USA e-mail: [email protected]
ROBERT S. COULTER*
Affiliation:
Department of Mathematical Sciences, University of Delaware, Newark, DE 19716, USA
BRADLEY FAIN
Affiliation:
6969 West 90th Ave, Apt 933, Westminster, CO 80021, USA e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For fixed m and a, we give an explicit description of those subsets of 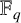${\mathbb F}_{q}$, q odd, for which both x and 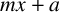$mx+a$ are quadratic residues (and other combinations). These results extend and refine results that date back to Gauss.

Keywords

quadratic residuesfinite fields

MSC classification

Primary: 11T30: Structure theory
Secondary: 11A07: Congruences; primitive roots; residue systems
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , First View , pp. 1 - 8
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

The second author gratefully acknowledges a bequest from the Estate of Francisco ‘Pancho’ Sayas, which partially supported this research.

References

Coulter, R. S. and Matthews, R. W., ‘On the permutation behaviour of Dickson polynomials of the second kind’, Finite Fields Appl. 8 (2002), 519–530.10.1016/S1071-5797(02)90361-8CrossRefGoogle Scholar
Davenport, H., The Higher Arithmetic, 8th edn (Cambridge University Press, Cambridge, 2008).10.1017/CBO9780511818097CrossRefGoogle Scholar
Dickson, L. E., Linear Groups with an Exposition of the Galois Field Theory (B. G. Teubner, Leipzig, 1901).Google Scholar
Henderson, M., ‘A note on the permutation behaviour of the Dickson polynomials of the second kind’, Bull. Aust. Math. Soc. 56 (1997), 499–505.10.1017/S0004972700031300CrossRefGoogle Scholar
Henderson, M. and Matthews, R., ‘Permutation properties of Chebyshev polynomials of the second kind over a finite field’, Finite Fields Appl. 1 (1995), 115–125.10.1006/ffta.1995.1008CrossRefGoogle Scholar
Henderson, M. and Matthews, R., ‘Dickson polynomials of the second kind which are permutation polynomials over a finite field’, New Zealand J. Math. 27 (1998), 227–244.Google Scholar
Nöbauer, W., ‘Über eine Klasse von Permutationspolynomen und die dadurch dargestellten Gruppen’, J. reine angew. Math. 231 (1968), 215–219.Google Scholar
Raber, N. C., ‘A geometric approach to counting distribution of squares in a finite field’, Geom. Dedicata 4 (1975), 297–303.10.1007/BF00148764CrossRefGoogle Scholar
Ralston, T., ‘On the distribution of squares in a finite field’, Geom. Dedicata 8 (1979), 207–212.CrossRefGoogle Scholar
Sun, Z.-H., ‘Consecutive numbers with the same Legendre symbol’, Proc. Amer. Math. Soc. 130 (2002), 2503–2507.CrossRefGoogle Scholar